Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-23T19:40:43.276Z Has data issue: false hasContentIssue false
  • English
  • Français

LITTLEWOOD–PALEY CHARACTERIZATION OF WEIGHTED HARDY SPACES ASSOCIATED WITH OPERATORS

Part of: Special classes of linear operators Harmonic analysis in several variables

Published online by Cambridge University Press:  10 November 2016

GUORONG HU
GUORONG HU*
Affiliation:
Department of Mathematics, Jiangxi Normal University, Nanchang, Jiangxi 330022, PR China email [email protected], [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 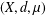$(X,d,\unicode[STIX]{x1D707})$ be a metric measure space endowed with a distance 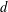$d$ and a nonnegative, Borel, doubling measure 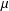$\unicode[STIX]{x1D707}$. Let $L$ be a nonnegative self-adjoint operator on $L^{2}(X)$. Assume that the (heat) kernel associated to the semigroup $e^{-tL}$ satisfies a Gaussian upper bound. In this paper, we prove that for any 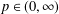$p\in (0,\infty )$ and 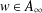$w\in A_{\infty }$, the weighted Hardy space 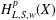$H_{L,S,w}^{p}(X)$ associated with $L$ in terms of the Lusin (area) function and the weighted Hardy space 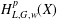$H_{L,G,w}^{p}(X)$ associated with 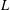$L$ in terms of the Littlewood–Paley function coincide and their norms are equivalent. This improves a recent result of Duong et al. [‘A Littlewood–Paley type decomposition and weighted Hardy spaces associated with operators’, J. Geom. Anal.26 (2016), 1617–1646], who proved that 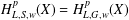$H_{L,S,w}^{p}(X)=H_{L,G,w}^{p}(X)$ for 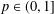$p\in (0,1]$ and 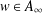$w\in A_{\infty }$ by imposing an extra assumption of a Moser-type boundedness condition on 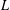$L$. Our result is new even in the unweighted setting, that is, when 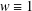$w\equiv 1$.

Keywords

Hardy spacesweightsnonnegative self-adjoint operatorsheat semigroupLusin and Littlewood–Paley functionsspace of homogeneous type

MSC classification

Primary: 42B25: Maximal functions, Littlewood-Paley theory
Secondary: 42B30: $H^p$-spaces 42B35: Function spaces arising in harmonic analysis 47B25: Symmetric and selfadjoint operators (unbounded)
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 103 , Issue 2 , October 2017 , pp. 250 - 267
Copyright
© 2016 Australian Mathematical Publishing Association Inc. 

References

Auscher, P., Duong, X. T. and McIntosh, A., ‘Boundedness of Banach space valued singular integral operators and Hardy spaces’, Unpublished manuscript, 2004.Google Scholar
Auscher, P., McIntosh, A. and Russ, E., ‘Hardy spaces of differential forms on Riemannian manifolds’, J. Geom. Anal. 18 (2008), 192–248.CrossRefGoogle Scholar
Auscher, P. and Russ, E., ‘Hardy spaces and divergence operators on strongly Lipschitz domains of ℝ n ’, J. Funct. Anal. 201 (2003), 148–184.CrossRefGoogle Scholar
Bui, T. A. and Duong, X. T., ‘Weighted Hardy spaces associated with operators and boundedness of singular integrals’, Preprint, 2012, arXiv:1202.2063.Google Scholar
Bui, H.-Q., Paluszyński, M. and Taibleson, M. H., ‘A maximal function characterization of Besov–Lipschitz and Triebel–Lizorkin spaces’, Studia Math. 119 (1996), 219–246.Google Scholar
Bui, H.-Q., Paluszyński, M. and Taibleson, M. H., ‘Characterization of the Besov–Lipschitz and Triebel–Lizorkin spaces. The case q < 1’, J. Fourier Anal. Appl. 3 (1997), 837–846.CrossRefGoogle Scholar
Coifman, R. R. and Weiss, G., ‘Extensions of Hardy spaces and their use in analysis’, Bull. Amer. Math. Soc. (N.S.) 83 (1977), 569–645.CrossRefGoogle Scholar
Duong, X. T. and Li, J., ‘Hardy spaces associated to operators satisfying Davies–Gaffney estimates and bounded holomorphic functional calculus’, J. Funct. Anal. 264 (2013), 1409–1437.CrossRefGoogle Scholar
Duong, X. T., Li, J. and Yan, L., ‘A Littlewood–Paley type decomposition and weighted Hardy spaces associated with operators’, J. Geom. Anal. 26 (2016), 1617–1646.CrossRefGoogle Scholar
Duong, X. T., Ouhabaz, E. M. and Sikora, A., ‘Plancherel-type estimates and sharp spectral multipliers’, J. Funct. Anal. 196 (2002), 443–485.CrossRefGoogle Scholar
Duong, X. T. and Yan, L., ‘Duality of Hardy and BMO spaces associated with operators with heat kernel bounds’, J. Amer. Math. Soc. 18 (2005), 943–973.CrossRefGoogle Scholar
Duong, X. T. and Yan, L., ‘New function spaces of BMO type, the John–Nirenberg inequality, interpolation, and applications’, Comm. Pure Appl. Math. 58 (2005), 1375–1420.CrossRefGoogle Scholar
Duong, X. T. and Yan, L., ‘Spectral multipliers for Hardy spaces associated to nonnegative self-adjoint operators satisfying Davies–Gaffney estimates’, J. Math. Soc. Japan 63 (2011), 295–319.CrossRefGoogle Scholar
Dziubański, J. and Preisner, M., ‘On Riesz transforms characterization of H 1 spaces associated with some Schrödinger operators’, Potential Anal. 35 (2011), 39–50.CrossRefGoogle Scholar
Dziubański, J. and Zienkiewicz, J., ‘Hardy space H 1 associated to Schrödinger operator with potential satisfying reverse Hölder inequality’, Rev. Mat. Iberoam. 15 (1999), 279–296.CrossRefGoogle Scholar
Dziubański, J. and Zienkiewicz, J., H p Spaces for Schrödinger Operators, Fourier Analysis and Related Topics (Bpolhk edlewo, 2000), Banach Center Publications, 56 (Polish Academy of Sciences, Warsaw, 2002), 45–53.Google Scholar
Garcia-Cuerva, J., ‘Weighted H p spaces’, Dissertationes Math. 162 (1979), 1–63.Google Scholar
Genebashvili, I., Gogatishvili, A., Kokilashvili, V. and Krbec, M., Weight Theory for Integral Transforms on Spaces of Homogeneous Type (Longman, Harlow, 1998).Google Scholar
Gong, R. and Yan, L., ‘Littlewood–Paley and spectral multipliers on weighted L p spaces’, J. Geom. Anal. 24 (2014), 873–900.CrossRefGoogle Scholar
Hofmann, S., Lu, G., Mitrea, D., Mitrea, M. and Yan, L., ‘Hardy spaces associated to non-negative self-adjoint operators satisfying Davies–Gaffney estimates’, Mem. Amer. Math. Soc. 214 (2011), 1–78.Google Scholar
Hofmann, S. and Mayboroda, S., ‘Hardy and BMO spaces associated to divergence form elliptic operators’, Math. Ann. 344 (2009), 37–116.CrossRefGoogle Scholar
Jiang, R. and Yang, D., ‘New Orlicz–Hardy spaces associated with divergence form elliptic operators’, J. Funct. Anal. 258 (2010), 1167–1224.CrossRefGoogle Scholar
Jiang, R. and Yang, D., ‘Orlicz–Hardy spaces associated with operators satisfying Davies–Gaffney estimates’, Commun. Contemp. Math. 13 (2011), 331–373.CrossRefGoogle Scholar
Ouhabaz, E. M., Analysis of Heat Equations on Domains, London Mathematical Society Monographs, 31 (Princeton University Press, Princeton, NJ, 2005).Google Scholar
Rychkov, V. S., ‘On a theorem of Bui, Paluszyński and Taibleson’, Proc. Steklov Inst. 227 (1999), 280–292.Google Scholar
Song, L. and Yan, L., ‘Riesz transforms associated to Schrödinger operators on weighted Hardy spaces’, J. Funct. Anal. 259 (2010), 1466–1490.CrossRefGoogle Scholar
Stein, E. M., Singular Integrals and Differentiability Properties of Functions, Princeton Mathematical Series, 30 (Princeton University Press, Princeton, NJ, 1970).Google Scholar
Strömberg, J.-O. and Torchinsky, A., Weighted Hardy Spaces, Lecture Notes in Mathematics, 1381 (Springer, Berlin, 1989).CrossRefGoogle Scholar
Wu, S., ‘A wavelet characterization for weighted Hardy spaces’, Rev. Mat. Iberoam. 8 (1992), 329–349.CrossRefGoogle Scholar
Yan, L., ‘Classes of Hardy spaces associated with operators, duality theory and applications’, Trans. Amer. Math. Soc. 360 (2008), 4383–4408.CrossRefGoogle Scholar