Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Jakubowski, Zbigniew Jerzy
1985.
On the properties of an integral operator.
Bulletin of the Australian Mathematical Society,
Vol. 32,
Issue. 1,
p.
55.
Kumar, Vinod
and
Shukla, S. L.
1986.
On an integral operator for convex univalent functions.
Bulletin of the Australian Mathematical Society,
Vol. 34,
Issue. 2,
p.
211.
Cho, Nak Eun
and
Owa, Shigeyoshi
2003.
Integral operators preserving certain analytic functions.
Journal of Mathematical Analysis and Applications,
Vol. 286,
Issue. 1,
p.
168.
Cho, Nak Eun
Bulboacă, Teodor
and
Srivastava, H.M.
2012.
A General family of integral operators and associated subordination and superordination properties of some special analytic function classes.
Applied Mathematics and Computation,
Vol. 219,
Issue. 4,
p.
2278.
Cho, Nak Eun
and
Bulboacă, Teodor
2013.
A class of integral operators preserving subordination and superordination.
Complex Variables and Elliptic Equations,
Vol. 58,
Issue. 7,
p.
909.
Cho, Nak Eun
and
Srivastava, H.M.
2023.
Subordinations by η-convex functions for a class of nonlinear integral operators.
Bulletin des Sciences Mathématiques,
Vol. 187,
Issue. ,
p.
103304.


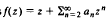 regular and satisfying │
regular and satisfying │