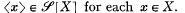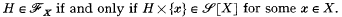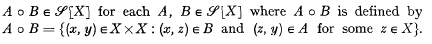Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Magill, Kenneth D.
1970.
Composable topological properties and semigroups of relations.
Journal of the Australian Mathematical Society,
Vol. 11,
Issue. 3,
p.
265.
Magill, K. D.
and
Yamamuro, S.
1971.
Union and symmetry preserving homomorphisms of semigroups of closed relations.
Semigroup Forum,
Vol. 3,
Issue. 1,
p.
168.
McAlister, D. B.
1971.
Homomorphisms of semigroups of binary relations.
Semigroup Forum,
Vol. 3,
Issue. 1,
p.
185.
Magill, Kenneth D
1972.
An abstract characterization of the semigroup of all closed relations on a c-space.
Journal of Mathematical Analysis and Applications,
Vol. 39,
Issue. 2,
p.
427.
Muenzenberger, T. B.
and
Smithson, R. E.
1974.
Compactness and connectedness as composable properties.
Journal of the Australian Mathematical Society,
Vol. 18,
Issue. 2,
p.
161.
O'Reilly, Sean B.
1975.
The characteristic semigroup of a topological space.
General Topology and its Applications,
Vol. 5,
Issue. 2,
p.
95.
Adu, David I.
1976.
The partially ordered family of CM-homomorphisms.
Semigroup Forum,
Vol. 13,
Issue. 1,
p.
1.
Adu, David I.
1977.
Existence of joins and meets in the partially ordered family of CM-homomorphisms.
Semigroup Forum,
Vol. 14,
Issue. 1,
p.
331.
Adu, David I.
1978.
Connected cm-homomorphisms into semigroups of closed binary relations on hereditarily locally connected continua.
Semigroup Forum,
Vol. 16,
Issue. 1,
p.
403.
Bandelt, Hans-J.
1981.
Isomorphisms between semigroups of isotone maps.
Journal of the Australian Mathematical Society,
Vol. 30,
Issue. 4,
p.
453.
Vechtomov, E. M.
1991.
Specification of topological spaces by algebraic systems of continuous functions.
Journal of Soviet Mathematics,
Vol. 53,
Issue. 2,
p.
123.
Varankina, V I
and
Vechtomov, Evgeniy Mikhailovich
2023.
Полугруппы относительно непрерывных бинарных отношений и их изоморфизмы.
Математические заметки,
Vol. 113,
Issue. 6,
p.
807.
Vechtomov, E. M.
2023.
Isomorphisms of semirings of continuous binary relations on topological spaces.
Semigroup Forum,
Vol. 106,
Issue. 1,
p.
327.
Varankina, V. I.
and
Vechtomov, E. M.
2023.
Semigroups of Relatively Continuous Binary Relations and Their Isomorphisms.
Mathematical Notes,
Vol. 113,
Issue. 5-6,
p.
760.