Published online by Cambridge University Press: 31 March 2014
Groebner bases for the ideals determining mod 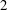 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}2$ cohomology of the real flag manifolds
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}2$ cohomology of the real flag manifolds 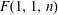 $F(1,1,n)$ and
$F(1,1,n)$ and  $F(1,2,n)$ are obtained. These are used to compute appropriate Stiefel–Whitney classes in order to establish some new nonembedding and nonimmersion results for the manifolds
$F(1,2,n)$ are obtained. These are used to compute appropriate Stiefel–Whitney classes in order to establish some new nonembedding and nonimmersion results for the manifolds 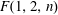 $F(1,2,n)$.
$F(1,2,n)$.