Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Vajner, Václav
1976.
Epeirogeny, orogeny and the tectonic development of the Kaapvaal basin, Southern Africa.
Geologische Rundschau,
Vol. 65,
Issue. 1,
p.
333.
Brandl, Rolf
1983.
Infinite soluble groups with engel cycles; A finiteness condition.
Mathematische Zeitschrift,
Vol. 182,
Issue. 2,
p.
259.


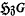 of the group
of the group 