No CrossRef data available.
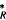 (M, M)
(M, M)Published online by Cambridge University Press: 09 April 2009
For a module M Over an Artin algebra R, we discuss the question of whether the Yoneda extension algebra Ext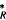 (M, M) is finitely generated as an algebra. We give an answer for bounded modules M. (These are modules whose syzygies have direct summands of bounded lengths.)
(M, M) is finitely generated as an algebra. We give an answer for bounded modules M. (These are modules whose syzygies have direct summands of bounded lengths.)