In [2] we studied parametric n-surfaces (f, Mn), where Mn was a compact, oriented, topological n-manifold and f a continuous mapping of Mn into the real euclidean k-space Rn (k≧n). A definition of bounded variation was given and, for each surface with bounded variation and each projection P from Rk to Rn, a signed measure: 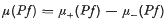Was constructed. This measure was used to define a linear type of surface integral: over a “measurable” subset A of Mn, as the Lebesgue-Stieltjes integral: .