Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-04T21:06:15.521Z Has data issue: false hasContentIssue false
  • English
  • Français

CONTINUED FRACTIONS FOR A CLASS OF TRIANGLE GROUPS

Part of: Diophantine approximation, transcendental number theory Other groups of matrices Probabilistic theory: distribution modulo $1$; metric theory of algorithms

Published online by Cambridge University Press:  07 February 2013

KARIANE CALTA  and
THOMAS A. SCHMIDT
KARIANE CALTA
Affiliation:
Vassar College, Poughkeepsie, NY 12604-0257, USA email [email protected]
THOMAS A. SCHMIDT*
Affiliation:
Oregon State University, Corvallis, OR 97331, USA
*
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

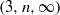We give continued fraction algorithms for each conjugacy class of triangle Fuchsian group of signature $(3, n, \infty )$, with 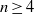$n\geq 4$. In particular, we give an explicit form of the group that is a subgroup of the Hilbert modular group of its trace field and provide an interval map that is piecewise linear fractional, given in terms of group elements. Using natural extensions, we find an ergodic invariant measure for the interval map. We also study Diophantine properties of approximation in terms of the continued fractions and show that these continued fractions are appropriate to obtain transcendence results.

Keywords

continued fractionsFuchsian groupsDiophantine approximation

MSC classification

Secondary: 11J70: Continued fractions and generalizations 11K50: Metric theory of continued fractions 11J17: Approximation by numbers from a fixed field 11J81: Transcendence (general theory) 20H10: Fuchsian groups and their generalizations
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 93 , Issue 1-2 , October 2012 , pp. 21 - 42
Copyright
Copyright ©2013 Australian Mathematical Publishing Association Inc.

References

Adamczewski, B. and Bugeaud, Y., ‘On the complexity of algebraic numbers II: Continued fractions’, Acta Math. 195 (2005), 1–20.CrossRefGoogle Scholar
Adamczewski, B. and Bugeaud, Y., ‘On the Maillet-Baker continued fractions’, J. reine angew. Math. 606 (2007), 105–121.Google Scholar
Adler, R., ‘Continued fractions and Bernoulli trials’, in: Ergodic Theory: A Seminar, Lecture Notes, 110 (eds. Moser, J., Phillips, E. and Varadhan, S.) (Courant Institute of Mathematical Sciences, New York University, New York, 1975).Google Scholar
Arnoux, P. and Hubert, P., ‘Fractions continuées sur les surfaces de Veech’, J. Anal. Math. 81 (2000), 35–64.CrossRefGoogle Scholar
Arnoux, P. and Schmidt, T. A., ‘Veech surfaces with nonperiodic directions in the trace field’, J. Mod. Dyn. 3 (4) (2009), 611–629.CrossRefGoogle Scholar
Baker, A., ‘Continued fractions of transcendental numbers’, Mathematika 9 (1962), 1–8.CrossRefGoogle Scholar
Barrionuevo, J., Burton, R., Dajani, K. and Kraaikamp, C., ‘Ergodic properties of generalized Lüroth series’, Acta Arith. 74 (4) (1996), 311–327.CrossRefGoogle Scholar
Borel, É., ‘Contribution à l’analyse arithmétique du continu’, J. Math. Pures Appl. 9 (1903), 329–375.Google Scholar
Bosma, W., Jager, H. and Wiedijk, F., ‘Some metrical observations on the approximation by continued fractions’, Indag. Math. 45 (3) (1983), 281–299.CrossRefGoogle Scholar
Bugeaud, Y., Approximation by Algebraic Numbers, Cambridge Tracts in Mathematics, 160 (Cambridge University Press, Cambridge, 2004).CrossRefGoogle Scholar
Bugeaud, Y., Hubert, P. and Schmidt, T. A., ‘Transcendence with Rosen continued fractions’, J. Eur. Math. Soc. (JEMS) 15 (2013), 39–51.CrossRefGoogle Scholar
Burton, R. M., Kraaikamp, C. and Schmidt, T. A., ‘Natural extensions for the Rosen fractions’, Trans. Amer. Math. Soc. 352 (1999), 1277–1298.CrossRefGoogle Scholar
Calta, K. and Smillie, J., ‘Algebraically periodic translation surfaces’, J. Mod. Dyn. 2 (2) (2008), 209–248.CrossRefGoogle Scholar
Cohen, P. and Wolfart, J., ‘Modular embeddings for some nonarithmetic Fuchsian groups’, Acta Arith. 56 (2) (1990), 93–110.CrossRefGoogle Scholar
Dajani, K., Kraaikamp, C. and Steiner, W., ‘Metrical theory for $\alpha $-Rosen fractions’, J. Eur. Math. Soc. (JEMS) 11 (6) (2009), 1259–1283.Google Scholar
Davenport, H. and Roth, K. F., ‘Rational approximations to algebraic numbers’, Mathematika 2 (1955), 160–167.CrossRefGoogle Scholar
Jager, H., ‘Continued fractions and ergodic theory’, in: Transcendental Number and Related Topics, RIMS Kokyuroku, 599 (Kyoto University, Kyoto, 1986), pp. 55–59.Google Scholar
Kenyon, R. and Smillie, J., ‘Billiards in rational-angled triangles’, Comment. Math. Helv. 75 (2000), 65–108.CrossRefGoogle Scholar
Kraaikamp, C., ‘A new class of continued fraction expansions’, Acta Arith. 57 (1) (1991), 1–39.CrossRefGoogle Scholar
Kraaikamp, C., Nakada, H. and Schmidt, T. A., ‘Metric and arithmetic properties of mediant-Rosen maps’, Acta Arith. 137 (4) (2009), 295–324.CrossRefGoogle Scholar
Kraaikamp, C. and Smeets, I., ‘Approximation results for $\alpha $-Rosen fractions’, Unif. Distrib. Theory 5 (2) (2010), 15–53.Google Scholar
Kraaikamp, C., Smeets, I. and Schmidt, T. A., ‘Tong’s spectrum for Rosen continued fractions’, J. Théor. Nombres Bordeaux 19 (3) (2007), 641–661.CrossRefGoogle Scholar
Maillet, E., Introduction à la théorie des nombres transcendants et des propriétés arithmétiques des fonctions (Gauthier-Villars, Paris, 1906).Google Scholar
Nakada, H., Ito, S. and Tanaka, S., ‘On the invariant measure for the transformations associated with some real continued-fractions’, Keio Engrg. Rep. 30 (13) (1977), 159–175.Google Scholar
Nakada, H., ‘On the Lenstra constant associated to the Rosen continued fractions’, J. Eur. Math. Soc. (JEMS) 12 (1) (2010), 55–70.CrossRefGoogle Scholar
Rohlin, V. A., ‘Exact endomorphisms of Lebesgue spaces’, Izv. Akad. Nauk SSSR Ser. Mat. 25 (1961), 499–530; Amer. Math. Soc. Transl., Series 2, 39 (1964), 1–36.Google Scholar
Rosen, D., ‘A class of continued fractions associated with certain properly discontinuous groups’, Duke Math. J. 21 (1954), 549–563.CrossRefGoogle Scholar
Schmutz Schaller, P. and Wolfart, J., ‘Semi-arithmetic Fuchsian groups and modular embeddings’, J. Lond. Math. Soc. 61 (1) (2000), 13–24.CrossRefGoogle Scholar
Schweiger, F., Ergodic Theory of Fibred Systems and Metric Number Theory (Clarendon Press, Oxford, 1995).Google Scholar
Smillie, J. and Ulcigrai, C., ‘Beyond Sturmian sequences: coding linear trajectories in the regular octagon’, Proc. Lond. Math. Soc. 102 (2011), 291–340.CrossRefGoogle Scholar
Smillie, J. and Ulcigrai, C., ‘Geodesic flow on the Teichmüller disk of the regular octagon, cutting sequences and octagon continued fractions maps’, in: Dynamical Numbers: Interplay Between Dynamical Systems and Number Theory, Contemporary Mathematics, 532 (American Mathematical Society, Providence, RI, 2010), pp. 29–65.CrossRefGoogle Scholar
Veech, W. A., ‘Teichmuller curves in modular space, Eisenstein series, and an application to triangular billiards’, Invent. Math. 97 (1989), 553–583.CrossRefGoogle Scholar
Ward, C., ‘Calculation of Fuchsian groups associated to billiards in a rational triangle’, Ergod. Theory Dynam. Sys. 18 (1998), 1019–1042.CrossRefGoogle Scholar