Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-05T01:02:00.506Z Has data issue: false hasContentIssue false
  • English
  • Français

COARSE AMENABILITY AND DISCRETENESS

Part of: Classical Topics in Algebraic Topology Special properties

Published online by Cambridge University Press:  21 October 2015

JERZY DYDAK
JERZY DYDAK*
Affiliation:
University of Tennessee, Knoxville, TN, USA email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

This paper is devoted to dualization of paracompactness to the coarse category via the concept of 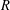$R$-disjointness. Property A of Yu can be seen as a coarse variant of amenability via partitions of unity and leads to a dualization of paracompactness via partitions of unity. On the other hand, finite decomposition complexity of Guentner, Tessera, and Yu and straight finite decomposition complexity of Dranishnikov and Zarichnyi employ 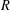$R$-disjointness as the main concept. We generalize both concepts to that of countable asymptotic dimension and our main result shows that it is a subclass of spaces with Property A. In addition, it gives a necessary and sufficient condition for spaces of countable asymptotic dimension to be of finite asymptotic dimension.

Keywords

absolute extensorsasymptotic dimensioncoarse geometrycoarse amenabilityLipschitz mapsProperty A

MSC classification

Primary: 54F45: Dimension theory
Secondary: 55M10: Dimension theory
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 100 , Issue 1 , February 2016 , pp. 65 - 77
Copyright
© 2015 Australian Mathematical Publishing Association Inc. 

References

Cencelj, M., Dydak, J. and Vavpetič, A., ‘Asymptotic dimension, Property A, and Lipschitz maps’, Rev. Mat. Complut. 26 (2013), 561–571.CrossRefGoogle Scholar
Cencelj, M., Dydak, J. and Vavpetič, A., ‘Large scale versus small scale’, in: Recent Progress in General Topology III (eds. Hart, K. P., van Mill, J. and Simon, P.) (Atlantis Press, Paris, 2014), 165–204.CrossRefGoogle Scholar
Cencelj, M., Dydak, J. and Vavpetič, A., ‘Coarse amenability versus paracompactness’, J. Topol. Anal. 6(1) (2014), 125–152.CrossRefGoogle Scholar
Dadarlat, M. and Guentner, E., ‘Uniform embeddability of relatively hyperbolic groups’, J. reine angew. Math. 612 (2007), 1–15.CrossRefGoogle Scholar
Dranishnikov, A., ‘Asymptotic topology’, Russian Math. Surveys 55(6) (2000), 1085–1129.CrossRefGoogle Scholar
Dranishnikov, A., Keesling, J. and Uspenskij, V. V., ‘On the Higson corona of uniformly contractible spaces’, Topology 37(4) (1998), 791–803.CrossRefGoogle Scholar
Dranishnikov, A. and Zarichnyi, M., ‘Asymptotic dimension, decomposition complexity, and Haver’s property C’, (2013) arXiv:1301.3484.Google Scholar
Dydak, J., ‘Extension theory: the interface between set-theoretic and algebraic topology’, Topology Appl. 20 (1996), 1–34.Google Scholar
Dydak, J. and Hoffland, C., ‘An alternative definition of coarse structures’, Topol. Appl. 155 (2008), 1013–1021.CrossRefGoogle Scholar
Dydak, J. and Mitra, A., ‘Large scale absolute extensors’, (2013) arXiv:1304.5987.Google Scholar
Engelking, R., General Topology (Heldermann, Berlin, 1989).Google Scholar
Gromov, M., ‘Asymptotic invariants for infinite groups’, in: Geometric Group Theory, 2 (eds. Niblo, G. and Roller, M.) (Cambridge University Press, Cambridge, 1993), 1–295.Google Scholar
Nowak, P. and Yu, G., Large Scale Geometry, EMS Textbooks in Mathematics (European Mathematical Society, Zurich, 2012).CrossRefGoogle Scholar
Ostrand, P. A., ‘Dimension of metric spaces and Hilbert’s problem 13’, Bull. Amer. Math. Soc. 71 (1965), 619–622.CrossRefGoogle Scholar
Ostrand, P., ‘A conjecture of J. Nagata on dimension and metrization’, Bull. Amer. Math. Soc. 71 (1965), 623–625.CrossRefGoogle Scholar
Ramras, D. A. and Ramsey, B. W., ‘Extending properties to relatively hyperbolic groups’, (2014)arXiv:1410.0060.Google Scholar
Roe, J., Lectures on Coarse Geometry, University Lecture Series, 31 (American Mathematical Society, Providence, RI, 2003).CrossRefGoogle Scholar
Willett, R., ‘Some notes on Property A’, in: Limits of Graphs in Group Theory and Computer Science (EPFL Press, Lausanne, 2009), 191–281.Google Scholar
Yu, G., ‘The coarse Baum–Connes conjecture for spaces which admit a uniform embedding into Hilbert space’, Invent. Math. 139 (2000), 201–240.CrossRefGoogle Scholar