Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-24T22:49:12.416Z Has data issue: false hasContentIssue false
  • English
  • Français

Classes of operators on vector valued integration spaces

Part of: Special classes of linear operators Linear function spaces and their duals

Published online by Cambridge University Press:  09 April 2009

R. J. Fleming  and
J. E. Jamison
J. E. Jamison
Affiliation:
Department of Mathematics, Memphis State University Memphis Tennessee 38152 USA
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

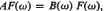Let Lp(Ω, K) denote the Banach space of weakly measurable functions F defined on a finite measure space and taking values in a separable Hilbert space K for which ∥ F ∥p = ( ∫ | F(ω) |p)1/p < + ∞. The bounded Hermitian operators on Lp(Ω, K) (in the sense of Lumer) are shown to be of the form , where B(ω) is a uniformly bounded Hermitian operator valued function on K. This extends the result known for classical Lp spaces. Further, this characterization is utilized to obtain a new proof of Cambern's theorem describing the surjective isometries of Lp(Ω, K). In addition, it is shown that every adjoint abelian operator on Lp(Ω, K) is scalar.

MSC classification

Secondary: 46E40: Spaces of vector- and operator-valued functions 46E30: Spaces of measurable functions ($L^p$-spaces, Orlicz spaces, Köthe function spaces, Lorentz spaces, rearrangement invariant spaces, ideal spaces, etc.) 47B99: None of the above, but in this section
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 24 , Issue 2 , September 1977 , pp. 129 - 138
Copyright
Copyright © Australian Mathematical Society 1977

References

Berkson, E. and Sourour, A. (1974), “The hermitian operators on some Banach spaces”, Studia Math. 52, 33–41.CrossRefGoogle Scholar
Cambern, M. (1974), “The isometries of Lp(X, K)”, Pacific J. Math. 55, 9–17.CrossRefGoogle Scholar
Dunford, N. and Schwartz, J. T. (1958), Linear Operators, Part 1 (Interscience, New York).Google Scholar
Fleming, R. J. and Jamison, J. E. (1974a), “Hermitian and adjoint abelian operators on certain Banach spaces”, Pacific J. Math. 52 (1), 67–84.CrossRefGoogle Scholar
Fleming, R. J. and Jamison, J. E. (1974b), “Isometries of certain Banach spaces”, J. London Math. Soc. (2), 9, 121–127.CrossRefGoogle Scholar
Fleming, R. J. and Jamison, J. E. (1976), “Adjoint abelian operators on Lp and C(K)”, Trans. Amer. Math. 217, 87–98.Google Scholar
Hille, E. and Phillips, R. (1957), Functional Analysis and Semi-groups (Colloquium Publications, Vol. 31, Providence, R.I.).Google Scholar
Koehler, D. and Rosenthal, P. (1970), “On isometries of normed linear spaces”, Studia Math. 38, 215–218.Google Scholar
Lamperti, J. (1958), “On the isometries of certain function spaces”, Pacific J. Math. 2, 459–466.CrossRefGoogle Scholar
Lang, S. (1969), Analysis II (Addison-Wesley, Reading, Mass.).Google Scholar
Lumer, G. (1961), “Semi-inner-Product Spaces”, Trans. Amer. Math. Soc. 100, 26–43.CrossRefGoogle Scholar
Lumer, G. (1963), “Isometries of reflexive Orlicz spaces”, Ann. Inst. Fourier, Grenoble, 13, 99–109.CrossRefGoogle Scholar
Stampfli, J. G. (1969), “Adjoint Abelian operators on Banach spaces”, Canadian J. Math. 31, 505–512.CrossRefGoogle Scholar
Tam, K. W. (1969), “Isometries of certain function spaces”, Pacific J. Math. 31, 233–246.CrossRefGoogle Scholar