No CrossRef data available.
Article contents
A characterization of the semigroup of matrix units
Published online by Cambridge University Press: 09 April 2009
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Let I be a set and let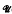 
(I) denote the set consisting of the 0 matrix over I × I and the matrix units over I × I. Then for x, z in 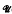(I) and x≠0≠z, xyz≠0 has precisely one solution y. This and several other statements are shown to be equivalent characterizations of 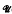(I) regarded as a semigroup with zero.
1980 Mathematics subject classification (Amer. Math. Soc.): 20 M 10.
- Type
- Research Article
- Information
- Copyright
- Copyright © Australian Mathematical Society 1980
You have
Access