Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Passi, I.B.S.
1975.
Annihilators of relation modules – II.
Journal of Pure and Applied Algebra,
Vol. 6,
Issue. 3,
p.
235.
Gupta, N.D
and
Passi, I.B.S
1976.
Some properties of fox subgroups of free groups.
Journal of Algebra,
Vol. 43,
Issue. 1,
p.
198.
Gupta, Narain D
Laffey, Thomas J
and
Thomson, Michael W
1979.
On the higher relation modules of a finite group.
Journal of Algebra,
Vol. 59,
Issue. 1,
p.
172.
Noskov, G. A.
Remeslennikov, V. N.
and
Roman'kov, V. A.
1982.
Infinite groups.
Journal of Soviet Mathematics,
Vol. 18,
Issue. 5,
p.
669.
MIKHAILOV, ROMAN
and
PASSI, INDER BIR S.
2006.
FAITHFULNESS OF CERTAIN MODULES AND RESIDUAL NILPOTENCE OF GROUPS.
International Journal of Algebra and Computation,
Vol. 16,
Issue. 03,
p.
525.
Mikhailov, R. V.
2006.
Faithful group actions and aspherical complexes.
Proceedings of the Steklov Institute of Mathematics,
Vol. 252,
Issue. 1,
p.
172.
Yamin, Mohammad
and
Sharma, P. K.
2014.
International Conference on Mathematical Sciences and Statistics 2013.
p.
265.
Yamin, Mohammad
and
Sharma, Poonam Kumar
2014.
RELATIVE RELATION MODULES OF FINITE ELEMENTARY ABELIAN p-GROUPS.
Bulletin of the Korean Mathematical Society,
Vol. 51,
Issue. 4,
p.
1205.


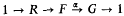 be a non-cyclic free presentation of a group
be a non-cyclic free presentation of a group 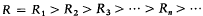 the lower central series of
the lower central series of 