Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-24T23:12:34.676Z Has data issue: false hasContentIssue false
  • English
  • Français

Analysis of the effect of certain forcings on the nonoscillatory solutions of even order equations

Part of: Qualitative theory

Published online by Cambridge University Press:  09 April 2009

Athanassios G. Kartsatos
Athanassios G. Kartsatos
Affiliation:
Department of Mathematics, University of South Florida Tampa Florida 33620 USA
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Properties of solutions of 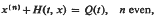, are studied in the cause uH(t, u)>0 for u≠0. It is shown that two inequalities may always be associated with (I) in such a way that if one of these inequalities has a small positive solution and the other inequality has a small negative solution, then (I) is oscillatory. Further asymptotic properties of (I) are studied under assumptions involving intermediate antiderivatives P(i)(t), with P(n) = Q. Several results of this type ensure the non-existence of positive solutions of (I).

MSC classification

Secondary: 34C10: Oscillation theory, zeros, disconjugacy and comparison theory
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 24 , Issue 2 , September 1977 , pp. 234 - 244
Copyright
Copyright © Australian Mathematical Society 1977

References

Atkinson, F. V. (1972/3), “On second order differential inequalities”, Proc. Royal Soc. Edinburgh, 72, 109–127.CrossRefGoogle Scholar
Bhatia, N. P. (1966), “Some oscillation theorems for second order differential equations”, J. Math. Anal. Appl. 15, 442–446.CrossRefGoogle Scholar
Foster, K. (1976a), “Oscillations of forced equations”, J. Differential Equations 20, 115–132.CrossRefGoogle Scholar
Foster, K. (1976b), “Oscillations of forced sublinear differential equations of even order”, J. Math. Anal. Appl 55, 634–643.CrossRefGoogle Scholar
Graef, J. R. (to appear), “Oscillation, nonoscillation, and growth of solutions of nonlinear functional differential equations of arbitrary order”.Google Scholar
Graef, J. R. and Spikes, P. W. (to appear), “Asymptotic properties of solutions of functional differential equations of arbitrary order”, J. Math. Anal. Appl.Google Scholar
Hammett, M. E. (1971), “Nonoscillation properties of a nonlinear differential equation”, Proc. AMS, 30, 92–96.CrossRefGoogle Scholar
Kartsatos, A. G. (1971), “On the maintenance of oscillations of nth order equations under the effect of a small forcing term”, J. Diff. Equations 10, 355–363.CrossRefGoogle Scholar
Kartsatos, A. G. (1972), “Maintenance of oscillations under the effect of a periodic forcing term”, Proc. AMS, 34, 377–383.CrossRefGoogle Scholar
Kartsatos, A. G. (1975), “On nth order differential inequalities”, J. Math. Anal. Appl. 52, 1–9.CrossRefGoogle Scholar
Kartsatos, A. G. (to appear), “Recent results on oscillation of solutions of forced and perturbed nonlinear equations of even order”, Proc. NSF-CBMS Reg. Conference, Stability of Dynamical Systems, Mississippi State Univ., Mississippi, edited by Graef, J. R. (Marcel Dekker, New York).Google Scholar
Kartsatos, A. G. (1976a), “Nth order oscillations with middle terms of order n−2”, Pacific J. Math. 67, 477–488.CrossRefGoogle Scholar
Kartsatos, A. G. (1976b), “Oscillation and existence of unique positive solutions for nonlinear nth order equations with forcing term”, Hiroshima Math. J. 6, 1–6.CrossRefGoogle Scholar
Kartsatos, A. G. (1976c), “On the oscillation problem of nonlinear equations”, Hiroshima Math. J. 6, 257–263.CrossRefGoogle Scholar
Kartsatos, A. G. and Manougian, M. N. (1974), “Perturbations causing oscillations of functional differential equations”, Proc. AMS 43, 111–117.CrossRefGoogle Scholar
Kartsatos, A. G. and Manougian, M. N. (1976), “Further results on oscillation of functional differential equations”, J. Math. Anal. Appl. 53, 28–37.CrossRefGoogle Scholar
Kusano, T. and Onose, H. (1974), “Oscillations of functional differential equations with retarded argument”, J. Diff. Equations 15, 269–277.CrossRefGoogle Scholar
Nehari, Z. (1963), “On a nonlinear differential equation arising in nuclear physics”, Proc. Royal Irish Acad. 62, 117–135.Google Scholar
Onose, H. (1973), “Oscillation and asymptotic behaviour of solutions of retarded differential equations of arbitrary order”, Hiroshima Math. J. 3, 333–360.CrossRefGoogle Scholar
Onose, H. (1975), “A comparison theorem and the forced oscillation”, Bull. Austral. Math. Soc. 13, 13–19.CrossRefGoogle Scholar
Staikos, V. A. and Sficas, Y. G. (1975a), “Forced oscillation for differential equations of arbitrary order”, J. Diff. Equations 17, 1–11.CrossRefGoogle Scholar
Staikos, V. A. and Sficas, Y. G. (1975b), “Oscillations for forced second order nonlinear differential equations”, Atti Acad. Naz. Lincei Rend. Cl. Sci. Fis. Mat. Natur. 58, 318–322.Google Scholar
Teufel, H. Jr, (1972), “Forced second order nonlinear oscillation”, J. Math. Anal. Appl. 40, 148–152.CrossRefGoogle Scholar
True, E. (1975), “A comparison theorem for certain functional differential equations”, Proc. AMS, 47, 127–132.CrossRefGoogle Scholar