No CrossRef data available.
Published online by Cambridge University Press: 21 December 2018
For an arbitrary discrete probability-measure-preserving groupoid 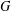 $G$, we provide a characterization of property (T) for
$G$, we provide a characterization of property (T) for  $G$ in terms of the groupoid von Neumann algebra
$G$ in terms of the groupoid von Neumann algebra  $L(G)$. More generally, we obtain a characterization of relative property (T) for a subgroupoid
$L(G)$. More generally, we obtain a characterization of relative property (T) for a subgroupoid 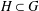 $H\subset G$ in terms of the inclusions
$H\subset G$ in terms of the inclusions 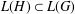 $L(H)\subset L(G)$.
$L(H)\subset L(G)$.
The author was partially supported by the NSF Grant DMS-1600186.