Hostname: page-component-669899f699-tpknm Total loading time: 0 Render date: 2025-04-25T04:56:33.587Z Has data issue: false hasContentIssue false
  • English
  • Français

SMALL VALUES AND FORBIDDEN VALUES FOR THE FOURIER ANTI-DIAGONAL CONSTANT OF A FINITE GROUP

Part of: Abstract harmonic analysis Representation theory of groups

Published online by Cambridge University Press:  07 April 2025

YEMON CHOI Open the ORCID record for YEMON CHOI [Opens in a new window]
YEMON CHOI*
Affiliation:
School of Mathematical Sciences, Lancaster University, Lancaster LA1 4YF, UK
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

For a finite group G, let $\operatorname { {AD}}(G)$ denote the Fourier norm of the antidiagonal in $G\times G$. The author showed recently in [‘An explicit minorant for the amenability constant of the Fourier algebra’, Int. Math. Res. Not. IMRN 2023 (2023), 19390–19430] that 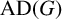$\operatorname { {AD}}(G)$ coincides with the amenability constant of the Fourier algebra of G and is equal to the normalized sum of the cubes of the character degrees of G. Motivated by a gap result for amenability constants from Johnson [‘Non-amenability of the Fourier algebra of a compact group’, J. Lond. Math. Soc. (2) 50 (1994), 361–374], we determine exactly which numbers in the interval $[1,2]$ arise as values of $\operatorname { {AD}}(G)$. As a by-product, we show that the set of values of 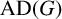$\operatorname { {AD}}(G)$ does not contain all its limit points. Some other calculations or bounds for $\operatorname { {AD}}(G)$ are given for familiar classes of finite groups. We also indicate a connection between 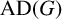$\operatorname { {AD}}(G)$ and the commuting probability of G, and use this to show that every finite group G satisfying 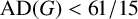$\operatorname { {AD}}(G)< {61}/{15}$ must be solvable; here, the value 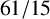${61}/{15}$ is the best possible.

Keywords

amenability constantcharacter theoryfinite groupFourier anti-diagonal constant

MSC classification

Primary: 20C15: Ordinary representations and characters
Secondary: 20D99: None of the above, but in this section 43A30: Fourier and Fourier-Stieltjes transforms on nonabelian groups and on semigroups, etc.
Type
Research Article
Information
Journal of the Australian Mathematical Society , First View , pp. 1 - 20
Check for updates
Copyright
© The Author(s), 2025. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

Footnotes

Communicated by Michael Giudici

References

Blichfeldt, H. F., ‘The finite, discontinuous primitive groups of collineations in four variables’, Math. Ann. 60 (1905), 204–231.CrossRefGoogle Scholar
Blichfeldt, H. F., ‘The finite, discontinuous, primitive groups of collineations in three variables’, Math. Ann. 63 (1907), 552–572.CrossRefGoogle Scholar
Choi, Y., ‘An explicit minorant for the amenability constant of the Fourier algebra’, Int. Math. Res. Not. IMRN 2023 (2023), 19390–19430.CrossRefGoogle Scholar
Choi, Y., ‘Moments of character degrees – is this result new or folklore?’ MathOverflow, 2023. URL: https://mathoverflow.net/q/437938 (version: 2023-01-08).Google Scholar
Gallagher, P. X., ‘The number of conjugacy classes in a finite group’, Math. Z. 118 (1970), 175–179.Google Scholar
Guralnick, R. M. and Robinson, G. R., ‘On the commuting probability in finite groups’, J. Algebra 300 (2006), 509–528.Google Scholar
James, G. and Liebeck, M., Representations and Characters of Groups, 2nd edn. (Cambridge University Press, New York, 2001).CrossRefGoogle Scholar
Johnson, B. E., ‘Non-amenability of the Fourier algebra of a compact group’, J. Lond. Math. Soc. (2) 50 (1994), 361–374.Google Scholar
Kovács, L. G. and Robinson, G. R., ‘On the number of conjugacy classes of a finite group’, J. Algebra 160 (1993), 441–460.CrossRefGoogle Scholar
Lau, A. T.-M., Loy, R. J. and Willis, G. A., ‘Amenability of Banach and ${\mathrm{C}}^{\ast }$ -algebras on locally compact groups’, Studia Math. 119 (1996), 161–178.Google Scholar
Miller, G. A., ‘Groups whose commutator subgroups are of order two’, Amer. J. Math. 60 (1938), 101–106.CrossRefGoogle Scholar
Sanders, T., ‘Coset decision trees and the Fourier algebra’, J. Anal. Math. 144 (2021), 227–259.CrossRefGoogle Scholar
Tong-Viet, H. P., ‘On groups with large character degree sums’, Arch. Math. (Basel) 99 (2012), 401–405.CrossRefGoogle Scholar
Walter, M. E., ‘ ${\mathrm{W}}^{\ast }$ -algebras and nonabelian harmonic analysis’, J. Funct. Anal. 11 (1972), 17–38.CrossRefGoogle Scholar