No CrossRef data available.
Article contents
BOCHNER–RIESZ MEANS ON BLOCK-SOBOLEV SPACES IN COMPACT LIE GROUP
Published online by Cambridge University Press: 08 January 2020
Abstract
On a compact Lie group 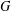 $G$ of dimension
$G$ of dimension  $n$, we study the Bochner–Riesz mean
$n$, we study the Bochner–Riesz mean 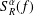 $S_{R}^{\unicode[STIX]{x1D6FC}}(f)$ of the Fourier series for a function
$S_{R}^{\unicode[STIX]{x1D6FC}}(f)$ of the Fourier series for a function 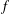 $f$. At the critical index
$f$. At the critical index  $\unicode[STIX]{x1D6FC}=(n-1)/2$, we obtain the convergence rate for
$\unicode[STIX]{x1D6FC}=(n-1)/2$, we obtain the convergence rate for 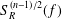 $S_{R}^{(n-1)/2}(f)$ when
$S_{R}^{(n-1)/2}(f)$ when 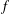 $f$ is a function in the block-Sobolev space. The main theorems extend some known results on the
$f$ is a function in the block-Sobolev space. The main theorems extend some known results on the  $m$-torus
$m$-torus 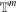 $\mathbb{T}^{m}$.
$\mathbb{T}^{m}$.
Keywords
- Type
- Research Article
- Information
- Journal of the Australian Mathematical Society , Volume 109 , Issue 2 , October 2020 , pp. 176 - 192
- Copyright
- © 2020 Australian Mathematical Publishing Association Inc.
Footnotes
The research was supported by National Natural Science Foundation of China (grant nos. 11671363, 11871436, 11871108, 11971295) and Natural Science Foundation of Shanghai (no. 19ZR1417600).



