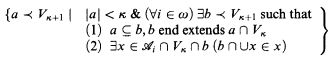Research Article
Defining relevant implication in a propositionally quantified S4
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1057-1069
-
- Article
- Export citation
More on imaginaries in p-adic fields
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1-13
-
- Article
- Export citation
Minimal invariant spaces in formal topology
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 689-698
-
- Article
- Export citation
Some applications of coarse inner model theory
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 337-365
-
- Article
- Export citation
A characterization of Martin's axiom in terms of absoluteness
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 366-372
-
- Article
- Export citation
Finitary sketches
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 699-707
-
- Article
- Export citation
T-convexity and tame extensions II
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 14-34
-
- Article
- Export citation
Amalgamations preserving ℵ1-categoricity
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1070-1074
-
- Article
- Export citation
Canonical seeds and Prikry trees
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 373-396
-
- Article
- Export citation
Lower bounds for cutting planes proofs with small coefficients
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 708-728
-
- Article
- Export citation
Splitting number at uncountable cardinals
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 35-42
-
- Article
- Export citation
A version of o-minimality for the p-adics
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1075-1092
-
- Article
- Export citation
Cardinal functions on ultra products of Boolean algebras
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 43-59
-
- Article
- Export citation
Generalized nonsplitting in the recursively enumerable degrees
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 397-437
-
- Article
- Export citation
Prototypes for definable subsets of algebraically closed valued fields
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1093-1141
-
- Article
- Export citation
Compact bracket abstraction in combinatory logic
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 729-740
-
- Article
- Export citation
Power types in explicit mathematics?
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 1142-1146
-
- Article
- Export citation
Precipitous towers of normal filters
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 741-754
-
- Article
- Export citation
Une correspondance entre anneaux partiels et groupes
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 60-78
-
- Article
- Export citation
The dense linear ordering principle
-
- Published online by Cambridge University Press:
- 12 March 2014, pp. 438-456
-
- Article
- Export citation

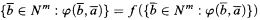
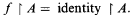

 determinacy implies that for every thin
determinacy implies that for every thin 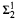 equivalence relation there is a
equivalence relation there is a 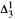 real,
real, 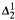 (
(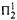 prewellorder has rank less than
prewellorder has rank less than 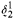 .
.
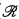

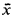
 → Γ be the associated valuation. “Definable” will mean “definable with parameters”.
→ Γ be the associated valuation. “Definable” will mean “definable with parameters”. 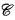 . Can we always find an amalgamation
. Can we always find an amalgamation 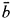 is sufficiently close to
is sufficiently close to 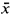 ,
, 

 , then
, then
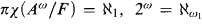 , where
, where 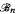 preserving (least and) greatest element, but also such that there is no (
preserving (least and) greatest element, but also such that there is no ( which has
which has 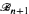 ). This theorem is the dual of a theorem of Ambos-Spies and Soare, and yields an alternative proof of their result that the theory of
). This theorem is the dual of a theorem of Ambos-Spies and Soare, and yields an alternative proof of their result that the theory of  are maximal antichains (
are maximal antichains (