Professor C. I. Lewis, in Lewis and Langford's Symbolic logic, designates the system (S2) determined by the postulates used in Chapter VI—namely, 11.1–7 (B1–7) and

as the system of strict implication. For certain reasons, he prefers it to either the earlier system (S3) determined by the stronger set of postulates of his Survey of symbolic logic, as emended, namely, A1–7 and

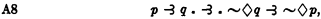
or the system (S1) determined by the weaker set of postulates B1–7 or A1–7.

But Lewis and others, following O. Becker, have also given consideration to systems which contain some additional principle effecting the reduction of complex modalities to simpler ones. Notable are the system (S4) determined by B1–7 plus

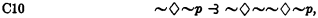
which includes (is stronger than) S3; and the system (S5) determined by B1–7 plus

which includes S4.

It seems worth while to investigate further the system of the Survey (emended), S3, which is intermediate between S2 and S4. This is the purpose of the present paper. We first prove some additional theorems in S2 and S3. These enable us to reduce all the complex modalities in S3 to a finite number, viz. 42; and it is shown that no further reduction is possible. Finally, several systems which include S3 are considered.

In this paper I shall show that no one of the four primitive symbols of Heyting's calculus of propositions is definable in terms of the other three. So as to make the paper self-contained, I begin by stating the rules and primitive sentences given by Heyting.

The primitive symbols of the calculus are “⅂”, “∨”, “∧”, and “⊃”, which may be read, respectively, as “not,” “either…or,” “and,” and “if…then.” The symbol “⊃⊂”, which may be read “if and only if,” is defined in terms of these as follows:

The rule of substitution is assumed, and the rule that S2 follows from S1 and S1⊃S2; in addition it is assumed that S1∧S2 follows from S1 and S2. The primitive sentences are as follows:

Let “the hypothesis of similarity” be the hypothesis that all infinite classes are similar (have the same power or cardinal number). It will be shown that the system of logic of Whitehead and Russell's Principia mathematica (without the axiom of reducibility) is consistent when to its axioms are added all the following:

(1). The hypothesis of similarity.

(2). The axiom of infinity.

(3). The axiom of choice.

(4). The contradictory of the axiom of reducibility.

If (2) – (4) but not (1) are employed in Principia, it must therefore be the case that Cantor's theorem is not deducible, since it contradicts (1). If (2) and (3) but not (1) and (4) are employed, then a proof of Cantor's theorem must require the use of some sort of reducibility principle.

The first step in the required consistency proof is to modify in certain respects the system S of a previous paper. Immediately after definition 3.5 the following definition is to be added:

3.5.1. If a is of the form (b,c), where x is an S-variable and where b and c are S-constants neither of which is of higher order than that of x, then a is an S-proposition.