Gödel introduced the original provability predicate in the proofs of Gödel’s incompleteness theorems, and Rosser defined a new one. They are equivalent in the standard model ${\mathbb N}$ of arithmetic or any nonstandard model of 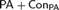${\rm PA} + {\rm Con_{PA}} $, but the behavior of Rosser’s provability predicate is different from the original one in nonstandard models of 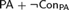${\rm PA} + \neg {\rm Con_{PA}} $. In this paper, we investigate several properties of the derivability conditions for Rosser provability predicates, and prove the existence of a Rosser provability predicate with which we can define any consistent complete extension of 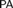${\rm PA}$ in some nonstandard model of 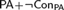${\rm PA} + \neg {\rm Con_{PA}} $. We call it a universal Rosser predicate. It follows from the theorem that the true arithmetic ${\rm TA}$ can be defined as the set of theorems of 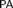${\rm PA}$ in terms of a universal Rosser predicate in some nonstandard model of 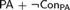${\rm PA} + \neg {\rm Con_{PA}} $. By using this theorem, we also give a new proof of a theorem that there is a nonstandard model M of ${\rm PA} + \neg {\rm Con_{PA}} $ such that if N is an initial segment of M which is a model of 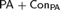${\rm PA} + {\rm Con_{PA}} $ then every theorem of 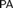${\rm PA}$ in N is a theorem of 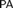$\rm PA$ in ${\mathbb N}$. In addition, we prove that there is a Rosser provability predicate such that the set of theorems of 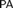$\rm PA$ in terms of the Rosser provability predicate is inconsistent in any nonstandard model of 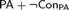${\rm PA} + \neg {\rm Con_{PA}} $.