Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-26T03:18:02.613Z Has data issue: false hasContentIssue false
  • English
  • Français

THE TOPOLOGICAL PIGEONHOLE PRINCIPLE FOR ORDINALS

Published online by Cambridge University Press:  29 June 2016

JACOB HILTON
JACOB HILTON*
Affiliation:
SCHOOL OF MATHEMATICS UNIVERSITY OF LEEDS LEEDS LS2 9JT, UKE-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Given a cardinal κ and a sequence 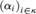${\left( {{\alpha _i}} \right)_{i \in \kappa }}$ of ordinals, we determine the least ordinal β (when one exists) such that the topological partition relation

$$\beta \to \left( {top\,{\alpha _i}} \right)_{i \in \kappa }^1$$
holds, including an independence result for one class of cases. Here the prefix “top” means that the homogeneous set must be of the correct homeomorphism class rather than the correct order type. The answer is linked to the nontopological pigeonhole principle of Milner and Rado.

Keywords

03E02pigeonhole principlepartition relationordinal topology
Type
Articles
Information
The Journal of Symbolic Logic , Volume 81 , Issue 2 , June 2016 , pp. 662 - 686
Copyright
Copyright © The Association for Symbolic Logic 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Baumgartner, James E, Partition relations for countable topological spaces . Journal of Combinatorial Theory, Series A, vol. 43(1986), no. 2, pp. 178–195.CrossRefGoogle Scholar
Friedman, Harvey, On closed sets of ordinals . Proceedings of the American Mathematical Society, vol. 43 (1974), no. 1, pp. 190–192.CrossRefGoogle Scholar
Milner, Eric C and Rado, Richard, The pigeon-hole principle for ordinal numbers . Proceedings of the London Mathematical Society, vol. 3 (1965), no. 1, pp. 750–768.CrossRefGoogle Scholar
Prikry, Karel and Solovay, Robert M, On partitions into stationary sets, this Journal, vol. 40 (1975), no. 1, pp. 75–80.Google Scholar
Shelah, Saharon, Proper and Improper Forcing, Springer, Berlin, 1998.CrossRefGoogle Scholar
Weiss, William, Partitioning topological spaces , Mathematics of Ramsey theory, pp. 154–171, Springer, Berlin, 1990.CrossRefGoogle Scholar