Article contents
TOPOLOGICAL COMPLETENESS OF LOGICS ABOVE S4
Published online by Cambridge University Press: 22 April 2015
Abstract
It is a celebrated result of McKinsey and Tarski [28] that S4 is the logic of the closure algebra Χ+ over any dense-in-itself separable metrizable space. In particular, S4 is the logic of the closure algebra over the reals R, the rationals Q, or the Cantor space C. By [5], each logic above S4 that has the finite model property is the logic of a subalgebra of Q+, as well as the logic of a subalgebra of C+. This is no longer true for R, and the main result of [5] states that each connected logic above S4 with the finite model property is the logic of a subalgebra of the closure algebra R+.
In this paper we extend these results to all logics above S4. Namely, for a normal modal logic L, we prove that the following conditions are equivalent: (i) L is above S4, (ii) L is the logic of a subalgebra of Q+, (iii) L is the logic of a subalgebra of C+. We introduce the concept of a well-connected logic above S4 and prove that the following conditions are equivalent: (i) L is a well-connected logic, (ii) L is the logic of a subalgebra of the closure algebra  $\xi _2^ + $ over the infinite binary tree, (iii) L is the logic of a subalgebra of the closure algebra
$\xi _2^ + $ over the infinite binary tree, (iii) L is the logic of a subalgebra of the closure algebra 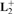 ${\bf{L}}_2^ + $ over the infinite binary tree with limits equipped with the Scott topology. Finally, we prove that a logic L above S4 is connected iff L is the logic of a subalgebra of R+, and transfer our results to the setting of intermediate logics.
${\bf{L}}_2^ + $ over the infinite binary tree with limits equipped with the Scott topology. Finally, we prove that a logic L above S4 is connected iff L is the logic of a subalgebra of R+, and transfer our results to the setting of intermediate logics.
Proving these general completeness results requires new tools. We introduce the countable general frame property (CGFP) and prove that each normal modal logic has the CGFP. We introduce general topological semantics for S4, which generalizes topological semantics the same way general frame semantics generalizes Kripke semantics. We prove that the categories of descriptive frames for S4 and descriptive spaces are isomorphic. It follows that every logic above S4 is complete with respect to the corresponding class of descriptive spaces. We provide several ways of realizing the infinite binary tree with limits, and prove that when equipped with the Scott topology, it is an interior image of both C and R. Finally, we introduce gluing of general spaces and prove that the space obtained by appropriate gluing involving certain quotients of L2 is an interior image of R.
Keywords
- Type
- Articles
- Information
- Copyright
- Copyright © The Association for Symbolic Logic 2015
References
REFERENCES
- 2
- Cited by


