Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-26T15:56:17.311Z Has data issue: false hasContentIssue false
  • English
  • Français

A PREDICATIVE VARIANT OF HYLAND’S EFFECTIVE TOPOS

Part of: Proof theory and constructive mathematics Computability and recursion theory Algebraic logic

Published online by Cambridge University Press:  22 October 2020

MARIA EMILIA MAIETTI  and
SAMUELE MASCHIO
MARIA EMILIA MAIETTI
Affiliation:
DIPARTIMENTO DI MATEMATICA “TULLIO LEVI-CIVITA” UNIVERSITÀ DI PADOVAPADOVA, ITALYE-mail:[email protected]:[email protected]
SAMUELE MASCHIO
Affiliation:
DIPARTIMENTO DI MATEMATICA “TULLIO LEVI-CIVITA” UNIVERSITÀ DI PADOVAPADOVA, ITALYE-mail:[email protected]:[email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Here, we present a category 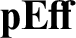${\mathbf {pEff}}$ which can be considered a predicative variant of Hyland's Effective Topos ${{\mathbf {Eff} }}$ for the following reasons. First, its construction is carried in Feferman’s predicative theory of non-iterative fixpoints 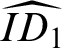${{\widehat {ID_1}}}$ . Second, ${\mathbf {pEff}}$ is a list-arithmetic locally cartesian closed pretopos with a full subcategory ${{\mathbf {pEff}_{set}}}$ of small objects having the same categorical structure which is preserved by the embedding in ${\mathbf {pEff}}$ ; furthermore subobjects in ${{\mathbf {pEff}_{set}}}$ are classified by a non-small object in ${\mathbf {pEff}}$ . 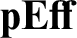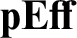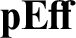Third ${\mathbf {pEff}}$ happens to coincide with the exact completion of the lex category defined as a predicative rendering in 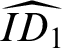${{\widehat {ID_1}}}$ of the subcategory of ${{\mathbf {Eff} }}$ of recursive functions and it validates the Formal Church’s thesis. Hence pEff turns out to be itself a predicative rendering of a full subcategory of ${{\mathbf {Eff} }}$ .

Keywords

toposesrecursion theorypredicative arithmeticsexact completionsintuitionistic logic

MSC classification

Primary: 03G30: Categorical logic, topoi
Secondary: 03D20: Recursive functions and relations, subrecursive hierarchies 03F55: Intuitionistic mathematics 03F30: First-order arithmetic and fragments
Type
Article
Information
The Journal of Symbolic Logic , Volume 86 , Issue 2 , June 2021 , pp. 433 - 447
Check for updates
Copyright
© The Association for Symbolic Logic 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aczel, P. and Rathjen, M., Notes on constructive set theory, Mittag-Leffler Technical report no. 40, 2001.Google Scholar
Carboni, A., Some free constructions in realizability and proof theory . Journal of Pure and Applied Algebra, vol. 103 (1995), pp. 117–148.CrossRefGoogle Scholar
Carboni, A. and Magno, R. C., The free exact category on a left exact one . Journal of the Australian Mathematical Society, vol. 33 (1982), pp. 295–301.10.1017/S1446788700018735CrossRefGoogle Scholar
Carboni, A. and Rosolini, G., Locally Cartesian closed exact completions . Journal of Pure and Applied Algebra, vol. 154 (2000), no. 1, pp. 103–116.CrossRefGoogle Scholar
Feferman, S., Iterated inductive fixed-point theories: Application to Hancock’s conjecture , Patras Logic Symposion (Metakides, G., editor), North Holland, Amsterdam, 1982, pp. 171–196.CrossRefGoogle Scholar
Ishihara, H., Maietti, M. E., Maschio, S. S., and Streicher, T., Consistency of the intensional level of the Minimalist Foundation with Church’s thesis and axiom of choice . Archive for Mathematical Logic, vol. 57 (2018), no. 7–8, pp. 873–888.CrossRefGoogle Scholar
Hyland, J. M. E., The effective topos , The LEJ Brouwer Centenary Symposium (Troelstra, A. S. and van Dalen, D., editors), Studies in Logic and the Foundations of Mathematics, vol. 110, North-Holland, Amsterdam, 1982, pp. 165–216.Google Scholar
Hyland, J. M. E., Johnstone, P. T., and Pitts, A. M., Tripos theory. Bulletin of the Australian Mathematical Society, vol. 88 (1980), pp. 205–232.Google Scholar
Maietti, M. E., A minimalist two-level foundation for constructive mathematics. Annals of Pure and Applied Logic, vol. 160 (2009), no. 3, pp. 319–354.CrossRefGoogle Scholar
Maietti, M. E., Joyal’s Arithmetic universe as list-arithmetic pretopos . Theory and Applications of Categories, vol. 24 (2010), no. 3, pp. 39–83.Google Scholar
Maietti, M. E., On choice rules in dependent type theory, Theory and Applications of Models of Computation (Gopal, T.V., Jäger, G., and Steila, S., editors), Springer, 2017, pp. 12– 23.CrossRefGoogle Scholar
Maietti, M. E. and Maschio, S., An extensional Kleene realizability semantics for the Minimalist Foundation, Proceedings of the 20th International Conference on Types for Proofs and Programs , LIPIcs. Leibniz International Proceedings in Informatics, vol. 39, Schloss Dagstuhl, Wadern, 2015, pp. 162–186.Google Scholar
Maietti, M. E. and Maschio, S., A predicative variant of a realizability tripos for the Minimalist Foundation . IfColog Journal of Logics and their Applications, vol. 3 (2016), no. 4, pp. 595–668.Google Scholar
Maietti, M. E., Pasquali, F., and Rosolini, G., Triposes, exact completions, and Hilbert’s ϵ-operator . Tbilisi Mathematical Journal, vol. 10 (2017), no. 3, pp. 141–166.CrossRefGoogle Scholar
Maietti, M. E., Pasquali, F., and Rosolini, G., Elementary quotient completions, Church’s thesis, and partioned assemblies . Logical Methods in Computer Science, vol. 15 (2019), no. 2, pp. 21:1-21:21.Google Scholar
Maietti, M. E. and Rosolini, G., Elementary quotient completion . Theory and Applications of Categories, vol. 27 (2013), no. 17, pp. 445–463.Google Scholar
Maietti, M. E. and Rosolini, G., Quotient completion for the foundation of constructive mathematics . Logica Universalis, vol. 7 (2013), no. 3, pp. 371–402.CrossRefGoogle Scholar
Maietti, M. E. and Rosolini, G., Relating quotient completions via categorical logic , Concepts of Proof in Mathematics, Philosophy, and Computer Science (Probst, D. and Schuster, P., editors), Walter de Gruyter, Berlin, 2016, p. 229.CrossRefGoogle Scholar
Maietti, M. E. and Sambin, G., Toward a minimalist foundation for constructive mathematics , From Sets and Types to Topology and Analysis: Practicable Foundations for Constructive Mathematics (Crosilla, L. and Schuster, P., editors), Oxford Logic Guides, vol. 48, Oxford University Press, Oxford, 2005, pp. 91–114.CrossRefGoogle Scholar
Nordström, B., Petersson, K., and Smith, J., Programming in Martin Löf’s Type Theory, Clarendon Press, Oxford, 1990.Google Scholar
Robinson, E. and Rosolini, G., Colimit completions and the effective topos, this Journal, vol. 55 (1990), no. 2, pp. 678–699.Google Scholar
van den Berg, B. and Moerdijk, I., Aspects of predicative algebraic set theory II: Realizability. Theoretical Computer Science, vol. 412 (2011), pp. 1916–1940.CrossRefGoogle Scholar
van Oosten, J., Axiomatizing higher order Kleene realizability. Annals of Pure and Applied Logic, vol. 70 (1994), no. 1, pp. 87–111.CrossRefGoogle Scholar
van Oosten, J., Realizability: A historical essay . Mathematical Structures in Computer Science, vol. 12 (2002), pp. 239–263.Google Scholar
van Oosten, J., Realizability: An Introduction to Its Categorical Side, Studies in Logic and Foundations of Mathematics, vol. 152, Elsevier, Amsterdam, 2008.Google Scholar