Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-31T19:32:54.158Z Has data issue: false hasContentIssue false
  • English
  • Français

P-hierarchy on βω

Published online by Cambridge University Press:  12 March 2014

Andrzej Starosolski
Andrzej Starosolski*
Affiliation:
Wydział Matematyczno-Fizyczny, Politechnika Ślaska, Gliwice, Poland, E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We classify ultrafilters on ω with respect to sequential contours (see [4]. [5]) of different ranks. In this way we obtain an ω1 sequence 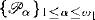of disjoint classes. We prove that non-emptiness of for successor α ≥ 2 is equivalent to the existence of P-point. We investigate relations between P-hierarchy and ordinal ultrafilters (introduced by J. E. Baumgartner in [1]). we prove that it is relatively consistent with ZFC that the successor classes (for α ≥ 2) of P-hierarchy and ordinal ultrafilters intersect but are not the same.

Keywords

P-hierarchyP-pointsmonotone sequential contourordinal ultrafilters
Type
Research Article
Information
The Journal of Symbolic Logic , Volume 73 , Issue 4 , December 2008 , pp. 1202 - 1214
Copyright
Copyright © Association for Symbolic Logic 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Baumgartner, J. E., Ultrafilters on ω, this Journal, vol. 60 (1995), no. 2, pp. 624–639.Google Scholar
[2]Comfort, W. W. and Negropontis, S., The theory of ultrafilters, Springer-Verlag, Berlin Heidelberg New York, 1974.CrossRefGoogle Scholar
[3]Dolecki, S., Multisequences, Quaestiones Mathematicae, vol. 29 (2007), pp. 239–277.CrossRefGoogle Scholar
[4]Dolecki, S. and Mynard, F., Cascades and multifilters, Topology and its Applications, vol. 104 (2002), pp. 53–65.CrossRefGoogle Scholar
[5]Dolecki, S., Starosolski, A., and Watson, S., Extension of multisequences and countable uniradial class of topologies, Commentationes Mathematicae Universitatis Carolinae, vol. 44 (2003), no. 1, pp. 165–181.Google Scholar
[6]Dolecki, S. and Watson, S., Mapping between Arens spaces, in Watson, S. homepeage: http://at.yorku.ca/z/a/a/a/12.htm.Google Scholar
[7]Frolík, Z., Sums of ultrafilters, Bulletin of the American Mathematical Society, vol. 73 (1967), pp. 87–91.CrossRefGoogle Scholar
[8]Grimeisen, G., Gefilterte Summation von Filtern und iterierte Grenzeprozesse, I, Mathematische Annalen, vol. 141 (1960), pp. 318–342.CrossRefGoogle Scholar
[9]Grimeisen, G., Gefilterte Summation von Filtern und iterierte Grenzeprozesse, II, Mathematische Annalen, vol. 144 (1961), pp. 386–417.CrossRefGoogle Scholar
[10]Laflamme, C., A few special ordinal ulteafilters, this Journal, vol. 61 (1996), no. 3, pp. 920–927.Google Scholar
[11]van Mill, J., An introduction to βω, Handbook of set-theoretic topology (Kunnen, K. and Vaughan, J. E., editors), North-Holland, 1988.Google Scholar
[12]Rudin, W., Homogeneity problems in the theory of čech compactyfications, Duke Mathematical Journal, vol. 23 (1956), pp. 409–419.CrossRefGoogle Scholar
[13]Shelah, S., Proper forcing, Lecture Notes in Mathematics 940, Springer-Verlag, 1982.CrossRefGoogle Scholar
[14]Starosolski, A., Fractal filters and supercontoures, Topology Proceedings, vol. 30 (2006), no. 1, pp. 389–402.Google Scholar
[15]Wimmets, E., Shelah P-point independence theorem, Israel Journal of Mathematics, vol. 43 (1982), pp. 28–48.CrossRefGoogle Scholar