Jónsson and Tarski [1951] introduced the notion of a Boolean algebra with (additive) operators (for short, a Bo). They showed that every Bo 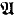can be extended to a complete and atomic Bo satisfying certain additional conditions, and that any two complete, atomic extensions of 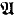satisfying these conditions are isomorphic over 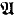. Henkin [1970] extended these results to Boolean algebras with generalized (i.e., weakly additive) operators. The particular complete, atomic extension of 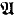studied by Jónsson and Tarski is called the perfect extension of 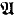, and is denoted by 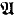+. It is very useful in algebraic investigations of classes of algebras that are associated with logics.

Interesting examples of Bos abound in algebraic logic, and include relation algebras, cylindric algebras, and polyadic and quasi-polyadic algebras (with or without equality). Moreover, there are several important constructions that, when applied to certain Bos, lead to other, derived Bos. Obvious examples include the formation of subalgebras, homomorphic images, relativizations, and direct products. Other examples include the Boolean algebra of ideal elements of a Bo, the neat β;-reduct of an α-dimensional cylindric algebra (β; < α), and the relation algebraic reduct of a cylindric algebra (of dimension at least 3). It is natural to ask about the relationship between the perfect extension of a Bo 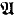and the perfect extension of one of its derived algebras 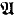′: Is the perfect extension of the derived algebra just the derived algebra of the perfect extension? In symbols, is (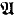′)+ = (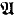+)′? For example, is the perfect extension of a subalgebra, homomorphic image, relativization, or direct product, just the corresponding subalgebra, homomorphic image, relativization, or direct product of the perfect extension (up to isomorphisms)? Is the perfect extension of the Boolean algebra of ideal elements, or the neat reduct of a cylindric algebra, or the relation algebraic reduct of a cylindric algebra just the Boolean algebra of ideal elements, or the neat β;-reduct, or the relation algebraic reduct, of the perfect extension? We shall prove a general result in this direction; namely, if the derived algebra is constructed as the range of a relatively multiplicative operator, then the answer to our question is “yes”. We shall also give examples to show that in “infinitary” constructions, our question can have a spectacularly negative answer.