Article contents
THE ONTO MAPPING OF SIERPINSKI AND NONMEAGER SETS
Published online by Cambridge University Press: 16 May 2017
Abstract
The principle (*) of Sierpinski is the assertion that there is a family of functions 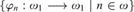 $\left\{ {{\varphi _n}:{\omega _1} \to {\omega _1}|n \in \omega } \right\}$ such that for every
$\left\{ {{\varphi _n}:{\omega _1} \to {\omega _1}|n \in \omega } \right\}$ such that for every  $I \in {[{\omega _1}]^{{\omega _1}}}$ there is n ε ω such that
$I \in {[{\omega _1}]^{{\omega _1}}}$ there is n ε ω such that 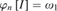 ${\varphi _n}[I] = {\omega _1}$. We prove that this principle holds if there is a nonmeager set of size ω1 answering question of Arnold W. Miller. Combining our result with a theorem of Miller it then follows that (*) is equivalent to
${\varphi _n}[I] = {\omega _1}$. We prove that this principle holds if there is a nonmeager set of size ω1 answering question of Arnold W. Miller. Combining our result with a theorem of Miller it then follows that (*) is equivalent to 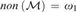 $non\left( {\cal M} \right) = {\omega _1}$. Miller also proved that the principle of Sierpinki is equivalent to the existence of a weak version of a Luzin set, we will construct a model where all of these sets are meager yet
$non\left( {\cal M} \right) = {\omega _1}$. Miller also proved that the principle of Sierpinki is equivalent to the existence of a weak version of a Luzin set, we will construct a model where all of these sets are meager yet 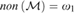 $non\left( {\cal M} \right) = {\omega _1}$.
$non\left( {\cal M} \right) = {\omega _1}$.
- Type
- Articles
- Information
- Copyright
- Copyright © The Association for Symbolic Logic 2017
References
REFERENCES
- 2
- Cited by


