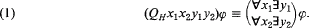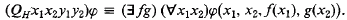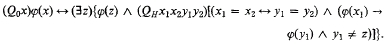Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Makowsky, J. A.
and
Shelah, S.
1979.
The theorems of Beth and Craig in abstract model theory. I. The abstract setting.
Transactions of the American Mathematical Society,
Vol. 256,
Issue. 0,
p.
215.
Väänänen, Jouko
1980.
Boolean valued models and generalized quantifiers.
Annals of Mathematical Logic,
Vol. 18,
Issue. 3,
p.
193.
Cowles, John R.
1981.
The Henkin Quantifier and Real Closed Fields.
Mathematical Logic Quarterly,
Vol. 27,
Issue. 31-35,
p.
549.
Ivanov, A. A.
1984.
Some theorems in generalized calculuses.
Siberian Mathematical Journal,
Vol. 24,
Issue. 6,
p.
868.
Mostowski, Marcin
1987.
Mathematical Logic and Its Applications.
p.
315.
Krynicki, Michal
1987.
On Some Applications of Games for Härtig Quantifier.
Mathematical Logic Quarterly,
Vol. 33,
Issue. 4,
p.
359.
Krynicki, Michael
and
Väänänen, Jouko
1989.
Henkin and function quantifiers.
Annals of Pure and Applied Logic,
Vol. 43,
Issue. 3,
p.
273.
Mostowski, Marcin
1989.
Pure Logic with Branched Quantifiers.
Mathematical Logic Quarterly,
Vol. 35,
Issue. 1,
p.
45.
Krynicki, Michał
and
Mostowski, Marcin
1992.
Decidability problems in languages with Henkin quantifiers.
Annals of Pure and Applied Logic,
Vol. 58,
Issue. 2,
p.
149.
Sandu, Gabriel
and
Väänänen, Jouko
1992.
PARTIALLY ORDERED CONNECTIVES.
Mathematical Logic Quarterly,
Vol. 38,
Issue. 1,
p.
361.
Sandu, Gabriel
1993.
On the logic of informational independence and its applications.
Journal of Philosophical Logic,
Vol. 22,
Issue. 1,
p.
29.
Krynicki, Michał
and
Mostowski, Marcin
1995.
Quantifiers: Logics, Models and Computation.
p.
193.
Hella, Lauri
and
Sandu, Gabriel
1995.
Quantifiers: Logics, Models and Computation.
p.
79.
Hintikka, Jaakko
1997.
Lingua Universalis vs. Calculus Ratiocinator.
p.
46.
Hintikka, Jaakko
and
Sandu, Gabriel
1997.
Handbook of Logic and Language.
p.
361.
Sandu, Gabriel
1998.
If-Logic and Truth-definition.
Journal of Philosophical Logic,
Vol. 27,
Issue. 2,
p.
143.
Hintikka, Jaakko
1998.
Language, Truth and Logic in Mathematics.
p.
189.
2003.
2002 European Summer Meeting of the Association for Symbolic Logic Logic Colloquium '02.
Bulletin of Symbolic Logic,
Vol. 9,
Issue. 1,
p.
71.
Mostowski, Marcin
and
Zdanowski, Konrad
2004.
Degrees of logics with Henkin quantifiers in poor vocabularies.
Archive for Mathematical Logic,
Vol. 43,
Issue. 5,
p.
691.
Gheerbrant, Amélie
and
Mostowski, Marcin
2006.
Recursive complexity of the Carnap first order modal logic C.
MLQ,
Vol. 52,
Issue. 1,
p.
87.