Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-22T20:28:08.822Z Has data issue: false hasContentIssue false
  • English
  • Français

On the ring of differentially-algebraic entire functions

Published online by Cambridge University Press:  12 March 2014

Lee A. Rubel
Lee A. Rubel*
Affiliation:
Department of Mathematics, University of Illinois, Urbana, Illinois 61801, E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Extract

Let 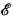be the ring of all entire functions of one complex variable, and let DA be the subring of those entire functions that are differentially algebraic (DA); that is, they satisfy a nontrivial algebraic differential equation.

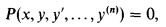
where P is a non-identically-zero polynomial in its n + 2 variables. It seems not to be known whether DA is elementarily equivalent to 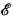. This would mean that DA and 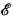have exactly the same true statements about them, in the first-order language of rings. (Roughly speaking, a sentence about a ring R is first-order if it has finite length and quantifies only over elements (i.e., not subsets or functions or relations) of R.) It follows from [NAN] that DA and 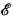are not isomorphic as rings, but this does not answer the question of elementary equivalence.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 57 , Issue 2 , June 1992 , pp. 449 - 451
Copyright
Copyright © Association for Symbolic Logic 1992

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[NAN]Nandakumar, N. R., Ring homomorphisms on algebras of analytic functions, preprint, 1990.Google Scholar
[BHR]Becker, J., Henson, C. W., and Rubel, L. A., First-order conformai invariants, Annals of Mathematics, ser. 2, vol. 112 (1980), pp. 123–178.CrossRefGoogle Scholar