No CrossRef data available.
Article contents
On the first-order logic of terms
Published online by Cambridge University Press: 12 March 2014
Extract
By an elementary condition in the variablesx1, …, xn, we mean a conjunction of the form x1 ≤ i < j ≤ naij where each aij is one of the formulas xi = xj or xi ≠ xj. (We should add that the formula x1 = x1 should be regarded as an elementary condition in the one variable x1.)
Clearly, according to this definition, some elementary conditions are inconsistent, some are consistent. For instance (in the variables x1, x2, x3) the conjunction x1 = x2 & x1 = x3 & x2 ≠ x3 is inconsistent.
By an elementary combinatorial function (ex. function) we mean any function which can be given a definition of the form

where E1(x1, …, xn), …, Ek(x1, …, xn) is an enumeration of all consistent elementary conditions in x1, …, xn, and all the numbers d1, …, dk are among 1, …, n.
Examples. (1) The identity function is the only 1-ary e.c. function.
(2) A useful 3-ary e.c. function will be called J. The definition is
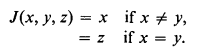
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1973


