Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-22T16:05:37.545Z Has data issue: false hasContentIssue false
  • English
  • Français

ON CATEGORICITY IN SUCCESSIVE CARDINALS

Part of: Model theory

Published online by Cambridge University Press:  20 July 2020

SEBASTIEN VASEY
SEBASTIEN VASEY*
Affiliation:
RADIX TRADING LLC. CHICAGO, IL60654, USAE-mail:[email protected]: svasey.github.io
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate, in ZFC, the behavior of abstract elementary classes (AECs) categorical in many successive small cardinals. We prove for example that a universal 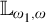$\mathbb {L}_{\omega _1, \omega }$ sentence categorical on an end segment of cardinals below $\beth _\omega $ must be categorical also everywhere above $\beth _\omega $ . This is done without any additional model-theoretic hypotheses (such as amalgamation or arbitrarily large models) and generalizes to the much broader framework of tame AECs with weak amalgamation and coherent sequences.

Keywords

abstract elementary classescategoricityamalgamationno maximal modelsgood frames

MSC classification

Primary: 03C48: Abstract elementary classes and related topics
Secondary: 03C45: Classification theory, stability and related concepts 03C52: Properties of classes of models 03C55: Set-theoretic model theory 03C75: Other infinitary logic
Type
Article
Information
The Journal of Symbolic Logic , Volume 87 , Issue 2 , June 2022 , pp. 545 - 563
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press on behalf of The Association for Symbolic Logic

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ackerman, N., Boney, W., and Vasey, S., Categoricity in multiuniversal classes . Annals of Pure and Applied Logic, vol. 170 (2019), no. 11. Article no. 102712.10.1016/j.apal.2019.06.001CrossRefGoogle Scholar
Baldwin, J. T., Categoricity, University Lecture Series, vol. 50, American Mathematical Society, Providence, RI, 2009.Google Scholar
Baldwin, J. T. and Kolesnikov, A., Categoricity, amalgamation, and tameness . Israel Journal of Mathematics, vol. 170 (2009), pp. 411–443.CrossRefGoogle Scholar
Boney, W., Grossberg, R., Vandieren, M., and Vasey, S., Superstability from categoricity in abstract elementary classes . Annals of Pure and Applied Logic, vol. 168 (2017), no. 7, pp. 1383–1395.10.1016/j.apal.2017.01.005CrossRefGoogle Scholar
Boney, W., Tameness and extending frames . Journal of Mathematical Logic, vol. 14 (2014), no. 2, Article no. 1450007.10.1142/S021906131450007XCrossRefGoogle Scholar
Baldwin, J. T. and Shelah, S., Examples of non-locality , this Journal, vol. 73 (2008), pp. 765–782.Google Scholar
Boney, W. and Vasey, S., A survey on tame abstract elementary classes , Beyond first order model theory (Iovino, J., editor), CRC Press, Boca Raton, FL, 2017, pp. 353–427.CrossRefGoogle Scholar
Boney, W. and Vasey, S., Tameness and frames revisited , this Journal, vol. 82 (2017), no. 3, pp. 995–1021.Google Scholar
Boney, W. and Vasey, S., Structural logic and abstract elementary classes with intersections . Bulletin of the Polish Academy of Science (Mathematics), vol. 67 (2019), pp. 1–17.10.4064/ba8178-12-2018CrossRefGoogle Scholar
Devlin, K. J. and Shelah, S., A weak version of  $\diamond$  which follows from  $2^{\aleph_0} < 2^{\aleph_1}$ . Israel Journal of Mathematics, vol. 29 (1978), no. 2, pp. 239–247.Google Scholar
Grossberg, R., Classification theory for abstract elementary classes . Contemporary Mathematics, vol. 302 (2002), pp. 165–204.10.1090/conm/302/05080CrossRefGoogle Scholar
Grossberg, R. and VanDieren, M., Categoricity from one successor cardinal in tame abstract elementary classes . Journal of Mathematical Logic, vol. 6 (2006), no. 2, pp. 181–201.10.1142/S0219061306000554CrossRefGoogle Scholar
Grossberg, R. and VanDieren, M., Galois-stability for tame abstract elementary classes . Journal of Mathematical Logic, vol. 6 (2006), no. 1, pp. 25–49.CrossRefGoogle Scholar
Grossberg, R. and VanDieren, M., Shelah's categoricity conjecture from a successor for tame abstract elementary classes , this Journal, vol. 71 (2006), no. 2, pp. 553–568.Google Scholar
Hart, B. and Shelah, S., Categoricity over $P$ for first order $T$ or categoricity for $\phi \in {L}_{\omega_1,\omega }$  can stop at  ${\aleph}_k$ while holding for  $\aleph_0, \aleph_{k-1}$ . Israel Journal of Mathematics, 70 (1990), 219–235.Google Scholar
Mazari-Armida, M. and Vasey, S., Universal classes near  $\aleph_1$ , this Journal, vol. 83 (2018), no. 4, pp. 1633–1643.Google Scholar
Rosický, J., Accessible categories, saturation and categoricity , this Journal, vol. 62 (1997), no. 3, pp. 891–901.Google Scholar
Shelah, S., Classification theory for non-elementary classes I: The number of uncountable models of $\psi \in {L}_{\omega_1,\omega }$ . Part A . Israel Journal of Mathematics, vol. 46 (1983), no. 3, pp. 214–240.Google Scholar
Shelah, S., Classification theory for non-elementary classes I: The number of uncountable models of $\psi \in {L}_{\omega_1,\omega }$ . Part B . Israel Journal of Mathematics, vol. 46 (1983), no. 4, pp. 241–273.10.1007/BF02762887CrossRefGoogle Scholar
Shelah, S., Classification of non elementary classes II. Abstract elementary classes, Classification Theory, (Baldwin, JT., editor), Lecture Notes in Mathematics, vol. 1292, Springer-Verlag, Berlin, 1987, pp.419–497.10.1007/BFb0082243CrossRefGoogle Scholar
Shelah, S., Universal classes , Classification Theory, (Baldwin, JT., editor), Lecture Notes in Mathematics, vol. 1292, Springer-Verlag, Berlin, 1987, pp. 264–418.CrossRefGoogle Scholar
Shelah, S., Categoricity for abstract classes with amalgamation . Annals of Pure and Applied Logic, vol. 98 (1999), no. 1, pp. 261–294.10.1016/S0168-0072(98)00016-5CrossRefGoogle Scholar
Shelah, S., Categoricity of an abstract elementary class in two successive cardinals . Israel Journal of Mathematics, vol. 126 (2001), pp. 29–128.CrossRefGoogle Scholar
Shelah, S., Classification theory for abstract elementary classes, Studies in Logic: Mathematical logic and foundations, vol. 18, College Publications, London, 2009.Google Scholar
Shelah, S., Classification theory for abstract elementary classes 2, Studies in Logic: Mathematical logic and foundations, vol. 20, College Publications, London, 2009.Google Scholar
Shelah, S. and Vasey, S., Categoricity and multidimensional diagrams, Preprint, 2018, https://arxiv.org/abs/1805.06291v2.Google Scholar
Shelah, S. and Villaveces, A., Toward categoricity for classes with no maximal models . Annals of Pure and Applied Logic, vol. 97 (1999), pp. 1–25.CrossRefGoogle Scholar
VanDieren, M., Categoricity in abstract elementary classes with no maximal models . Annals of Pure and Applied Logic, vol. 141 (2006), pp. 108–147.10.1016/j.apal.2005.10.006CrossRefGoogle Scholar
Vasey, S., Forking and superstability in tame AECs , this Journal, vol. 81 (2016), no. 1, pp. 357–383.Google Scholar
Vasey, S., Infinitary stability theory . Archive for Mathematical Logic, vol. 55 (2016), pp. 567–592.10.1007/s00153-016-0481-zCrossRefGoogle Scholar
Vasey, S., Saturation and solvability in abstract elementary classes with amalgamation . Archive for Mathematical Logic, vol. 56 (2017), pp. 671–690.CrossRefGoogle Scholar
Vasey, S., Shelah's eventual categoricity conjecture in universal classes: Part I . Annals of Pure and Applied Logic, vol. 168 (2017), no. 9, pp. 1609–1642.CrossRefGoogle Scholar
Vasey, S., Shelah's eventual categoricity conjecture in universal classes: Part II . Selecta Mathematica, vol. 23 (2017), no. 2, pp. 1469–1506.10.1007/s00029-016-0296-0CrossRefGoogle Scholar
Vasey, S., Toward a stability theory of tame abstract elementary classes . Journal of Mathematical Logic, vol. 18 (2018), no. 2, Article no. 1850009.10.1142/S0219061318500095CrossRefGoogle Scholar
Vasey, S., The categoricity spectrum of large abstract elementary classes with amalgamation . Selecta Mathematica, vol. 25 (2019), no. 5, p. 65.10.1007/s00029-019-0511-xCrossRefGoogle Scholar
Vasey, S., Tameness from two successive good frames . Israel Journal of Mathematics, vol. 235 (2020), pp. 465–500.10.1007/s11856-020-1965-4CrossRefGoogle Scholar
Zilber, B., A categoricity theorem for quasi-minimal excellent classes, logic and its applications , Contemporary Mathematics (Blass, A. and Zhang, Y., editors), American Mathematical Society, Providence, RI, 2005, pp. 297–306.CrossRefGoogle Scholar