Article contents
On atomic or saturated sets
Published online by Cambridge University Press: 12 March 2014
Abstract
Assume T is stable, small and Φ(x) is a formula of L(T). We study the impact on T⌈Φ of naming finitely many elements of a model of T. We consider the cases of T⌈Φ which is ω-stable or superstable of finite rank. In these cases we prove that if T has 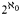 countable models and Q = Φ(M) is countable and atomic or saturated, then any good type in S(Q) is τ-stable. If T⌈Φ is ω-stable and (bounded, 1-based or of finite rank) with
countable models and Q = Φ(M) is countable and atomic or saturated, then any good type in S(Q) is τ-stable. If T⌈Φ is ω-stable and (bounded, 1-based or of finite rank) with  , then we prove that every good p ∈ S(Q) is τ-stable for any countable Q. The proofs of these results lead to several new properties of small stable theories, particularly of types of finite weight in such theories.
, then we prove that every good p ∈ S(Q) is τ-stable for any countable Q. The proofs of these results lead to several new properties of small stable theories, particularly of types of finite weight in such theories.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1996
References
REFERENCES
- 2
- Cited by


