Article contents
A NOTE ON DERIVABILITY CONDITIONS
Published online by Cambridge University Press: 07 September 2020
Abstract
We investigate relationships between versions of derivability conditions for provability predicates. We show several implications and non-implications between the conditions, and we discuss unprovability of consistency statements induced by derivability conditions. First, we classify already known versions of the second incompleteness theorem, and exhibit some new sets of conditions which are sufficient for unprovability of Hilbert–Bernays’ consistency statement. Secondly, we improve Buchholz’s schematic proof of provable 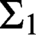 $\Sigma_1$
-completeness. Then among other things, we show that Hilbert–Bernays’ conditions and Löb’s conditions are mutually incomparable. We also show that neither Hilbert–Bernays’ conditions nor Löb’s conditions accomplish Gödel’s original statement of the second incompleteness theorem.
$\Sigma_1$
-completeness. Then among other things, we show that Hilbert–Bernays’ conditions and Löb’s conditions are mutually incomparable. We also show that neither Hilbert–Bernays’ conditions nor Löb’s conditions accomplish Gödel’s original statement of the second incompleteness theorem.
MSC classification
- Type
- Articles
- Information
- Copyright
- © The Association for Symbolic Logic 2020
References
REFERENCES
- 7
- Cited by



