Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-27T01:05:19.407Z Has data issue: false hasContentIssue false
  • English
  • Français

Models of arithmetic and closed ideals

Published online by Cambridge University Press:  12 March 2014

Julia Knight  and
Mark Nadel
Julia Knight
Affiliation:
University of Notre Dame, Notre Dame, Indiana 46556
Mark Nadel
Affiliation:
University of Notre Dame, Notre Dame, Indiana 46556
Get access Rights & Permissions [Opens in a new window]

Extract

A set J of Turing degrees is called an ideal if (1) J ≠ ∅, (2) for any pair of degrees ã, 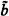, if ã, 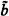ϵ J, then ã ⋃ 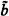ϵJ, and (3) for any ⋃ ϵ J and any 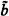, if 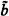< ⋃, then 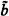ϵ J. A set J of degrees is said to be closed if for any theory T with a set of axioms of degree in J, T has a completion of degree in J.

Closed ideals of degrees arise naturally in the following way. If is a recursively saturated structure, let I() = { for some ā ϵ }. Let D() = {: is recursive in d-saturated}. (Recursive in d-saturation is defined like recursive saturation except that the sets of formulas considered are recursive in d.) These two sets of degrees were investigated in [2]. It was shown that if is a recursively saturated model of P, Pr = Th(ω, +), or Pr′ = Th(Z, +, 1), then I() = D(), and this set is a closed ideal. Any closed ideal J can be represented as I() = D() for some recursively saturated model of Pr′. For sets J of power at most ℵ1, Pr′ can be replaced by P.

Assuming CH, all closed ideals have power at most ℵ1, but if CH fails, there are closed ideals of power greater than ℵ1, and it is not known whether these can be represented as I() = D() for a recursively saturated model of P.

In the present paper, it will first be shown that information about representation of closed ideals provides new information about an old problem of MacDowell and Specker [6] and extends an old result of Scott [8] in a natural way. It will also be shown that the representation results from [2] answer a problem of Friedman [1]. This part of the paper is aimed at convincing the reader that representation problems are worth investigating.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 47 , Issue 4 , December 1982 , pp. 833 - 840
Copyright
Copyright © Association for Symbolic Logic 1982

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Friedman, H., Countable models of set theories, Lecture Notes in Mathematics, vol. 337, Springer-Verlag, Berlin and New York, 1973, pp. 539–573.Google Scholar
[2]Knight, J. and Nadel, M., Expansions of models and Turing degrees, this Journal, vol. 47 (1982), pp. 587–604.Google Scholar
[3]Knight, J., Amalgamation of recursively saturated structures (preprint).Google Scholar
[4]Knight, J., Additive structure in uncountable models for a fixed completion of P (preprint).Google Scholar
[5]Lipshitz, L. and Nadel, M., The additive structure of models of arithmetic, Proceedings of the American Mathematical Society, vol. 68 (1978), pp. 331–336.CrossRefGoogle Scholar
[6]MacDowell, R. and Specker, E., Modelle der Arithmetik, Infinitistic methods, Pergamon Press, London, 1961, pp. 257–263.Google Scholar
[7]Nadel, M., On a problem of MacDowell and Specker, this Journal, vol. 45 (1980), pp. 612–622.Google Scholar
[8]Scott, D., Algebras of sets binumerable in complete extensions of arithmetic, Proceedings of Symposia in Pure Mathematics, vol. 5, American Mathematical Society, Providence, R.I., 1962, pp. 117–121.Google Scholar