Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-31T18:44:09.806Z Has data issue: false hasContentIssue false
  • English
  • Français

Generic trees

Published online by Cambridge University Press:  12 March 2014

Otmar Spinas
Otmar Spinas*
Affiliation:
Mathematik Eth-Zentrum, 8092 Zürich, Switzerland Institute of Mathematics, The Hebrew University, Givat Ram, 91904 Jerusalem, Israel
*
Department of Mathematics, University of California, Irvine, California 92717, E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

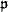We continue the investigation of the Laver ideal ℓ0 and Miller ideal m0 started in [GJSp] and [GRShSp]; these are the ideals on the Baire space associated with Laver forcing and Miller forcing. We solve several open problems from these papers. The main result is the construction of models for t < add(ℓ0), < add(m0), where add denotes the additivity coefficient of an ideal. For this we construct amoeba forcings for these forcings which do not add Cohen reals. We show that 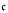= ω2 implies add(m0) ≤ 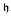. 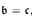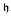We show that , implies cov(ℓ0) ≤ +, cov(m0) ≤ 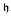+ respectively. Here cov denotes the covering coefficient. We also show that in the Cohen model cov(m0) < 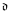holds. Finally we prove that Cohen forcing does not add a superperfect tree of Cohen reals.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 60 , Issue 3 , September 1995 , pp. 705 - 726
Copyright
Copyright © Association for Symbolic Logic 1995

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[BPS]Balcar, B., Pelant, J. and Simon, P., The space of ultrafilters on N covered by nowhere dense sets, Fundamenta Mathematicae, vol. 110 (1980), pp. 11–24.CrossRefGoogle Scholar
[BS]Balcar, B. and Simon, P., Disjoint refinement, Handbook of Boolean algebra, North-Holland, Amsterdam, 1989, pp. 333–386.Google Scholar
[BJ]Bartoszyński, T., Judah, H. and Shelah, S., The Cichoń diagram, this Journal, vol. 58 (1993), pp. 401–423.Google Scholar
[BD]Baumgartner, J. E. and Dordal, P., Adjoining dominating functions, this Journal, vol. 35 (1987), pp. 449–455.Google Scholar
[B]Bell, M. G., On the combinatorial principle P(c), Fundamenta Mathematicae, vol. 114 (1981), pp. 149–157.CrossRefGoogle Scholar
[Bl]Blass, A., Applications of superperfect forcing and its relatives, Set theory and its applications (Steprans, J. and Watson, S., editors), Lecture Notes in Mathematics, vol. 1401, Springer-Verlag, Berlin, 1989, pp. 18–40.CrossRefGoogle Scholar
[Br]Brendle, J., Mutually generic sets and perfect free subsets, preprint.Google Scholar
[vD]van Douwen, E. K., Integers and topology, Handbook of set theoretic topology (Kunen, K. and Vaughan, J., editors), North-Holland, Amsterdam, 1984, pp. 111–167.CrossRefGoogle Scholar
[E]Eisworth, T., Groupwise density and tree ideals, preprint.Google Scholar
[G]Goldstern, M., Tools for your forcing construction, Set theory of the reals (Ramat Gan, 1991), Israel Mathematical Conference Proceedings, vol. 6 (Judah, H., editor), Bar-Ilan University, Ramat Gan, 1993, pp. 305–360.Google Scholar
[GJSp]Goldstern, M., Johnson, M. and Spinas, O., Towers on trees, Proceedings of the American Mathematical Society, vol. 122 (1994), pp. 557–564.CrossRefGoogle Scholar
[GRShSp]Goldstern, M., Repický, M., Shelah, S. and Spinas, O., On tree ideals, Proceedings of the American Mathematical Society (to appear).Google Scholar
[JMSh]Judah, H., Miller, A. and Shelah, S., Sacks forcing, Laver forcing, and Martin's axiom, Archive for Mathematical Logic, vol. 31 (1992), pp. 145–161.CrossRefGoogle Scholar
[JSh]Judah, H. and Shelah, S., The Kunen-Miller chart, this Journal, vol. 55 (1990), pp. 909–927.Google Scholar
[K]Kechris, A., On a notion of smallnessfor subsets of the Baire space, Transactions of the American Mathematical Society, vol. 229 (1977), pp. 191–207.CrossRefGoogle Scholar
[L]Laver, R., On the consistency of Borel's conjecture, Acta Mathematica, vol. 137 (1976), pp. 151–169.CrossRefGoogle Scholar
[M]Marczewski, E., Sur une classe de fonctions de W. Sierpiński et la classe correspondante d'ensembles, Fundamenta Mathematicae, vol. 24 (1935), pp. 17–34.Google Scholar
[Mi]Miller, A., Rational perfect set forcing, Axiomatic set theory (Baumgartner, J. E.et al., editors), Contemporary Mathematics, vol. 31, American Mathematical Society, Providence, Rhode Island, 1984, pp. 143–159.CrossRefGoogle Scholar
[Pl]Plewik, S., On completely Ramsey sets, Fundamenta Mathematicae, vol. 127 (1986), pp. 127–132.CrossRefGoogle Scholar
[R]Repický, M., Handwritten notes.Google Scholar
[Sh]Shelah, S., Proper forcing, Lecture Notes in Mathematics, vol. 940, Springer-Verlag, Berlin, 1982.CrossRefGoogle Scholar
[V]Veličković, B., CCC posets of perfect trees, Compositio Mathematica, vol. 79 (1991), pp. 279–294.Google Scholar