Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-26T03:06:01.353Z Has data issue: false hasContentIssue false
  • English
  • Français

Fusion over sublanguages

Published online by Cambridge University Press:  12 March 2014

Assaf Hasson  and
Martin Hils
Assaf Hasson
Affiliation:
The Mathematical Institute, 24–29 St. Giles, Oxford, OX1 3LB, UK
Martin Hils
Affiliation:
Institut für Mathematik, Humboldt-Universitätzu Berlin, D-10099 Berlin, Germany
Get access Rights & Permissions [Opens in a new window]

Abstract

Generalising Hrushovski's fusion technique we construct the free fusion of two strongly minimal theories T1. T2 intersecting in a totally categorical sub-theory T0. We show that if. e.g., T0 is the theory of infinite vector spaces over a finite field then the fusion theory Tω, exists, is complete and ω-stable of rank ω. We give a detailed geometrical analysis of Tω, proving that if both T1, T2 are 1-based then. Tω can be collapsed into a strongly minimal theory, if some additional technical conditions hold—all trivially satisfied if T0 is the theory of infinite vector spaces over a finite field .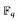

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 71 , Issue 2 , June 2006 , pp. 361 - 398
Copyright
Copyright © Association for Symbolic Logic 2006

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Baldwin, J. T., Fundamentals of stability theory, Springer Verlag. 1988.CrossRefGoogle Scholar
[2]Baldwin, J. T., Notes on quasiminimality and excellence, preprint, 2004.CrossRefGoogle Scholar
[3]Baldwin, J. T. and Holland, K., Constructing ω-stable structures: Rank 2 fields, this Journal, vol. 65 (2000), pp. 371–391.Google Scholar
[4]Baldwin, J. T. and Holland, K., Constructing ω-stable structures: Computing rank, Fundamenta Mathematkae. vol. 170 (2001). pp. 1–20.CrossRefGoogle Scholar
[5]Baldwin, J. T., Constructing ω-stable structures: Model completeness, Annals of Pure and Applied Logic, vol. 125 (2004), pp. 159–172.CrossRefGoogle Scholar
[6]Baudisch, A., Martin-Pizarro, A., and Ziegler, M., Fields and colors, preprint. 2005.Google Scholar
[7]Baudisch, A., Martin-Pizarro, A., and Ziegler, M., Fusion over a vector space, preprint. 2005.CrossRefGoogle Scholar
[8]Cherlin, G.. Harrington, L., and Lachlan, A.. ℵ0-Categorical. ℵ0-stable structures, Annals of Pure and Applied Logic, vol. 28 (1985), pp. 103–135.CrossRefGoogle Scholar
[9]Cherlin, G. and Hrushovski, E., Finite structures with few types, Annals of Mathematics Studies, vol. 152. Princeton University Press, 2003.Google Scholar
[10]Cohn, P. M., Skew field constructions, London Mathematical Society Lecture Note Series, vol. 27, Cambridge University Press, 1977.Google Scholar
[11]Gute, H. P. and Reuter, K. K.. The last word on elimination of quantifiers in modules, this JOurnal, vol. 55 (1990), pp. 670–673.Google Scholar
[12]Hasson, A.. Collapsing structure and a theory of envelopes, preprint. 2004.Google Scholar
[13]Hasson, A., Fusion over sublanguges revisited, preprint, 2006. (Available on author's webpage).Google Scholar
[14]Hils, M., La fusion au-dessus d'un sous-langage: le cas simple, preprint, 2004.Google Scholar
[15]Hils, M., Ph.D. thesis, in preperation.Google Scholar
[16]Holland, K., Model completeness of the new strongly minimal sets, this Journal, vol. 64 (1999), pp. 946–962.Google Scholar
[17]Hrushovski, E., Strongly minimal expansions of algebraically closed fields, Israel Journal of Mathematics, vol. 79 (1992). pp. 129–151.CrossRefGoogle Scholar
[18]Hrushovski, E., A new strongly minimal set, Annals of Pure and Applied Logic, vol. 62 (1993), pp. 147–166.CrossRefGoogle Scholar
[19]Pillay, A.. Geometric stability theory, Clarendon Press, Oxford, 1996.CrossRefGoogle Scholar
[20]Poizat, B., Le carré de l'égalité, this Journal, vol. 64 (1999), pp. 1339–1355.Google Scholar
[21]Poizat, B., L'égalité au cube, this Journal, vol. 66 (2001), pp. 1647–1676.Google Scholar
[22]Zil'ber, B., Uncountably categorical theories. Translations of Mathematical Monographs, vol. 117, American Mathematical Society, Providence, R.I., 1993.CrossRefGoogle Scholar