Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-31T19:52:56.967Z Has data issue: false hasContentIssue false
  • English
  • Français

Differentially algebraic group chunks

Published online by Cambridge University Press:  12 March 2014

Anand Pillay
Anand Pillay*
Affiliation:
Department of Mathematics, University of Notre Dame, Notre Dame, Indiana 46556
Get access Rights & Permissions [Opens in a new window]

Extract

We point out that a group first order definable in a differentially closed field K of characteristic 0 can be definably equipped with the structure of a differentially algebraic group over K. This is a translation into the framework of differentially closed fields of what is known for groups definable in algebraically closed fields (Weil's theorem).

I restrict myself here to showing (Theorem 20) how one can find a large “differentially algebraic group chunk” inside a group defined in a differentially closed field. The rest of the translation (Theorem 21) follows routinely, as in [B].

What is, perhaps, of interest is that the proof proceeds at a completely general (soft) model theoretic level, once Facts 1–4 below are known.

Fact 1. The theory of differentially closed fields of characteristic 0 is complete and has quantifier elimination in the language of differential fields (+, ·,0,1, −1,d).

Fact 2. Affine n-space over a differentially closed field is a Noetherian space when equipped with the differential Zariski topology.

Fact 3. If K is a differentially closed field, k ⊆ K a differential field, and a and 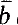are in k, then a is in the definable closure of k ◡ 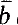iff a ∈ ‹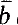› (where k ‹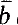› denotes the differential field generated by k and).

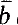Fact 4. The theory of differentially closed fields of characteristic zero is totally transcendental (in particular, stable).

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 55 , Issue 3 , September 1990 , pp. 1138 - 1142
Copyright
Copyright © Association for Symbolic Logic 1990

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[B]Bouscaren, E., Model theoretic versions of Weil's theorem on pregroups, The model theory of groups (stable group seminar, Notre Dame, Indiana, 1985–1987; Nesin, A. and Pillay, A., editors), Notre Dame Mathematical Lectures, vol. 11, Notre Dame University Press, Notre Dame, Indiana, 1989, pp. 177–185.Google Scholar
[K]Kolchin, E. R., Differentially algebraic groups, Academic Press, Orlando, Florida, 1985.Google Scholar
[PS]Pillay, A. and Srour, G., Closed sets and chain conditions in stable theories, this Journal, vol. 49 (1984), pp. 1350–1362.Google Scholar
[P]Poizat, B., Groupes stables, avec types génériques réguliers, this Journal, vol. 48 (1983), pp. 339–355.Google Scholar
[S]Sacks, G., Saturated model theory, Benjamin, Reading, Massachusetts, 1972.Google Scholar
[Sr]Srour, G., The notion of independence in categories of algebraic structures. Part I: Basic properties, Annals of Pure and Applied Logic, vol. 38 (1988), pp. 185–211.CrossRefGoogle Scholar
[W]Weil, A., On algebraic groups of transformations, American Journal of Mathematics, vol. 77 (1955), pp. 355–391.CrossRefGoogle Scholar