Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-22T23:17:51.409Z Has data issue: false hasContentIssue false
  • English
  • Français

A decidable subclass of the minimal Gödel class with identity

Published online by Cambridge University Press:  12 March 2014

Warren D. Goldfarb ,
Yuri Gurevich  and
Saharon Shelah
Warren D. Goldfarb
Affiliation:
Department of Philosophy, Harvard University, Cambridge, Massachusetts 02138
Yuri Gurevich
Affiliation:
Department of Computer and Communication Sciences, University of Michigan, Ann Arbor, Michigan 48104
Saharon Shelah
Affiliation:
Department of Mathematics, Hebrew University, Jerusalem, Israel
Get access Rights & Permissions [Opens in a new window]

Extract

The minimal Gödel class with identity (MGCI) is the class of closed, prenex quantificational formulas whose prefixes have the form ∀x1∀x2∃x3 and whose matrices contain arbitrary predicate letters and the identity sign “=”, but contain no function signs or individual constants. The MGCI was shown undecidable (for satisfiability) in 1983 [Go2]; this both refutes a claim of Gödel's [Gö, p. 443] and settles the decision problem for all prefix-classes of quantification theory with identity.

In this paper, we show the decidability of a natural subclass of the MGCI. The formulas in this subclass can be thought of as exploiting only half of the power of the existential quantifier. That is, since an MGCI formula has prefix ∀x1∀x2∃x3, in general its truth in a model requires for any elements a and b, the existence of both a witness for 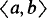and a witness for 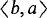. The formulas we consider demand less: they require, for any elements a and b, a witness for the unordered pair {a, b}, that is, a witness either for 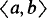or for .

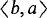Type
Research Article
Information
The Journal of Symbolic Logic , Volume 49 , Issue 4 , December 1984 , pp. 1253 - 1261
Copyright
Copyright © Association for Symbolic Logic 1984

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[CK]Chang, C. C. and Keisler, H. J., Model theory, North-Holland, Amsterdam, 1973.Google Scholar
[Go]Gödel, K., Zum Entscheidungsproblem des logischen Funktionenkalküls, Monatshefte für Mathematik und Physik, vol. 40 (1933), pp. 433–443.CrossRefGoogle Scholar
[Go1]Goldfarb, W. D., On the Gödel class with identity, this Journal, vol. 46 (1981), pp. 354–364.Google Scholar
[Go2]Goldfarb, W. D., The unsolvability of the Godel class with identity, this Journal, vol. 49 (1984), pp. 1237–1252.Google Scholar
[GS]Gurevich, Y. and Shelah, S., Random models and the Godel case of the decision problem, this Journal, vol. 48 (1983), pp. 1120–1124.Google Scholar
[KS]Ketonen, J. and Solovay, R., Rapidly growing Ramsey functions, Annals of Mathematics, ser. 2, vol. 113 (1981), pp. 267–314.CrossRefGoogle Scholar
[W]Wainer, S., A classification of the ordinal recursive functions, Archiv für mathematische Logik und Grundlagenforschung, vol. 13 (1970), pp. 136–153.CrossRefGoogle Scholar