Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-22T05:05:54.876Z Has data issue: false hasContentIssue false
  • English
  • Français

The club principle and the distributivity number

Published online by Cambridge University Press:  12 March 2014

Heike Mildenberger
Heike Mildenberger*
Affiliation:
Einstein Institute or Mathematics, The Hebrew University, Edmond Safra Campus Givat Ram, Jerusalem 91904, Israel, E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We give an affirmative answer to Brendle's and Hrušák's question of whether the club principle together with 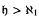is consistent. We work with a class of axiom A forcings with countable conditions such that 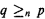is determined by finitely many elements in the conditions p and q and that all strengthenings of a condition are subsets, and replace many names by actual sets. There are two types of technique: one for tree-like forcings and one for forcings with creatures that are translated into trees. Both lead to new models of the club principle.

Keywords

Ostaszewski clubaxiom A forcingcardinal characteristics
Type
Research Article
Information
The Journal of Symbolic Logic , Volume 76 , Issue 1 , March 2011 , pp. 34 - 46
Copyright
Copyright © Association for Symbolic Logic 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Bartoszyński, Tomek and Judah, Haim, Set theory, on the structure of the real line, A K Peters, 1995.Google Scholar
[2]Baumgartner, James E. and Laver, Richard, Iterated perfect-set forcing, Annals of Mathematical Logic, vol. 17 (1979), pp. 271–288.CrossRefGoogle Scholar
[3]Blass, A., Combinatorial cardinal characteristics of the continuum, Handbook of set theory (Foreman, Matthew and Kanamori, Akihiro, editors), vol. 1, Springer, 2010, pp. 395–490.CrossRefGoogle Scholar
[4]Blass, Andreas, Applications of superperfect forcing and its relatives, Set theory and its applications (Steprāns, Juris and Watson, Steve, editors), Lecture Notes in Mathematics, vol. 1401, 1989, pp. 18–40.CrossRefGoogle Scholar
[5]Blass, Andreas and Shelah, Saharon, There may be simple Pℵ1- and Pℵ2-points and the Rudin-Keisler ordering may be downward directed, Annals of Pure and Applied Logic vol. 33 (1987), pp. 213–243.CrossRefGoogle Scholar
[6]Brendle, Jörg, Cardinal invariants of the continuum and combinatorics on uncountable cardinals, Annals of Pure and Applied Logic, vol. 144 (2006), pp. 43–72.CrossRefGoogle Scholar
[7]Broverman, Samuel, Ginsburg, John, Kunen, Kenneth, and Tall, Franklin, Topologies determined by σ-ideals on ω1, Canadian Journal of Mathematics, vol. 30 (1978), pp. 1306–1312.CrossRefGoogle Scholar
[8]Devlin, Keith J. and Shelah, Saharon, A weak version of ♢ which follows from 2ℵ0 < 2ℵ1, Israel Journal of Mathematics, vol. 29 (1978), pp. 239–247.CrossRefGoogle Scholar
[9]Džamonja, Mirna and Shelah, Saharon, ♣ does not imply the existence of a Suslin tree, Israel Journal of Mathematics, vol. 113 (1999), pp. 163–204.CrossRefGoogle Scholar
[10]Fuchino, Sakaé, Mildenberger, Heike, Shelah, Saharon, and Vojtáš, Peter, On absolutely divergent series, Fundamenta Mathematicae, vol. 160 (1999), pp. 255–268.CrossRefGoogle Scholar
[11]Fuchino, Sakaé, Shelah, Saharon, and Soukup, Lajos, Sticks and clubs, Annals of Pure and Applied Logic, vol. 90 (1997), pp. 57–77, math.LO/9804153.CrossRefGoogle Scholar
[12]Hrušák, Michal, Life in the Sacks model, Acta Universitatis Carolinae. Mathematica et Physica, vol. 42 (2001), pp. 43–58.Google Scholar
[13]Jech, Thomas, Set theory, Springer, 2003, the Third Millenium Edition, revised and expanded.Google Scholar
[14]Moore, Justin Tatch, Hrušák, Michael, and Džamonja, Mirna, Parametrized ♢ principles, Transactions of the American Mathematical Society, vol. 356 (2004), pp. 2281–2306.CrossRefGoogle Scholar
[15]Ostaszewski, A. J., A perfectly normal countably compact scattered space which is not strongly zero-dimensional, Journal of the London Mathematical Society. Second Series, vol. 14 (1976), no. 1, pp. 167–177.CrossRefGoogle Scholar
[16]Rosłanowski, Andrzej and Shelah, Saharon, Norms on possibilities. I. Forcing with trees and creatures, Memoirs of the American Mathematical Society, vol. 141 (AMS, 1999), no. 671.Google Scholar
[17]Shelah, Saharon, Proper and improper forcing, 2nd ed., Springer, 1998.CrossRefGoogle Scholar
[18]Truss, J. K., The noncommutativity of random and generic extensions, this Journal, vol. 48 (1983), no. 4, pp. 1008–1012.Google Scholar