If F is a field, and α is an element of F, we say that α is arithmetically definable in F if there is a formula containing one free variable and any number of bound variables, involving only the concepts of elementary logic and the operations of addition and multiplication, which is satisfied by α and by no other element of F. The range of the bound variables is understood to be F. Without changing the sense of the above definition, we can allow in our formulas symbols for specific integers, or even (if F has characteristic zero) symbols for specific rational numbers, since these are arithmetically definable.

As an example, consider the field F = R(2¼), obtained by adjoining the positive fourth root of 2 to the field R of rationals. Notice that 2¼ is not defined arithmetically by the formula x2 = 2, since this equation has two roots in F.

However, 2¼ may be defined arithmetically by the equivalence

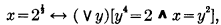
where we have used the logical symbols ↔ (if and only if), ∨ (there exists), and ∧ (and). For the equation y4 = 2 is satisfied by no elements of F except y = ±2¼, and in both cases y2 = 2¼. On the other hand, 2¼ is not arithmetically definable in F, since there is an automorphism of F which takes 2¼ into −2¼, so that every arithmetical condition satisfied by 2¼ is also satisfied by −2¼.

In any field F, a necessary condition for the arithmetical definability of an element α is that α should be fixed for all automorphisms of F. That this condition is not always sufficient is shown by considering the field of real numbers. Here there is no automorphism but the identity, but there can of course be but a denumerable infinity of arithmetically definable real numbers. Tarski has shown that only the algebraic numbers are arithmetically definable.