Article contents
WHAT THE ŁUKASIEWICZ AXIOMS MEAN
Published online by Cambridge University Press: 30 October 2020
Abstract
Let 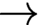 $\to $
be a continuous
$\to $
be a continuous  $\protect \operatorname {\mathrm {[0,1]}}$
-valued function defined on the unit square
$\protect \operatorname {\mathrm {[0,1]}}$
-valued function defined on the unit square  $\protect \operatorname {\mathrm {[0,1]}}^2$
, having the following properties: (i)
$\protect \operatorname {\mathrm {[0,1]}}^2$
, having the following properties: (i)  $x\to (y\to z)= y\to (x\to z)$
and (ii)
$x\to (y\to z)= y\to (x\to z)$
and (ii)  $x\to y=1 $
iff
$x\to y=1 $
iff 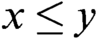 $x\leq y$
. Let
$x\leq y$
. Let 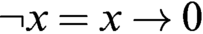 $\neg x=x\to 0$
. Then the algebra
$\neg x=x\to 0$
. Then the algebra 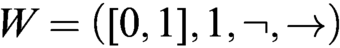 $W=(\protect \operatorname {\mathrm {[0,1]}},1,\neg ,\to )$
satisfies the time-honored Łukasiewicz axioms of his infinite-valued calculus. Let
$W=(\protect \operatorname {\mathrm {[0,1]}},1,\neg ,\to )$
satisfies the time-honored Łukasiewicz axioms of his infinite-valued calculus. Let 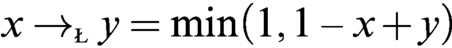 $x\to _{\text {\tiny \L }}y=\min (1,1-x+y)$
and
$x\to _{\text {\tiny \L }}y=\min (1,1-x+y)$
and 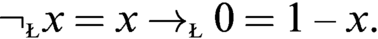 $\neg _{\text {\tiny \L }}x=x\to _{\text {\tiny \L }} 0 =1-x.$
Then there is precisely one isomorphism
$\neg _{\text {\tiny \L }}x=x\to _{\text {\tiny \L }} 0 =1-x.$
Then there is precisely one isomorphism 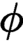 $\phi $
of W onto the standard Wajsberg algebra
$\phi $
of W onto the standard Wajsberg algebra 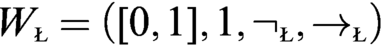 $W_{\text {\tiny \L }}= (\protect \operatorname {\mathrm {[0,1]}},1,\neg _{\text {\tiny \L }},\to _{\text {\tiny \L }})$
. Thus
$W_{\text {\tiny \L }}= (\protect \operatorname {\mathrm {[0,1]}},1,\neg _{\text {\tiny \L }},\to _{\text {\tiny \L }})$
. Thus 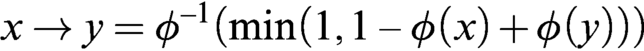 $x\to y= \phi ^{-1}(\min (1,1-\phi (x)+\phi (y)))$
.
$x\to y= \phi ^{-1}(\min (1,1-\phi (x)+\phi (y)))$
.
Keywords
- Type
- Articles
- Information
- Copyright
- © The Association for Symbolic Logic 2020
References
REFERENCES
- 3
- Cited by



