Published online by Cambridge University Press: 29 August 2019
We prove that the Wadge order on the Borel subsets of the Scott domain is not a well-quasi-order, and that this feature even occurs among the sets of Borel rank at most 2. For this purpose, a specific class of countable 2-colored posets  $\mathbb{P}_{emb} $ equipped with the order induced by homomorphisms is embedded into the Wadge order on the
$\mathbb{P}_{emb} $ equipped with the order induced by homomorphisms is embedded into the Wadge order on the  $\Delta _2^0 $-degrees of the Scott domain. We then show that
$\Delta _2^0 $-degrees of the Scott domain. We then show that 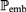 $\mathbb{P}_{emb} $ admits both infinite strictly decreasing chains and infinite antichains with respect to this notion of comparison, which therefore transfers to the Wadge order on the
$\mathbb{P}_{emb} $ admits both infinite strictly decreasing chains and infinite antichains with respect to this notion of comparison, which therefore transfers to the Wadge order on the  $\Delta _2^0 $-degrees of the Scott domain.
$\Delta _2^0 $-degrees of the Scott domain.