Published online by Cambridge University Press: 23 October 2018
In this article the lightface 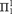 ${\rm{\Pi }}_1^1$-Comprehension axiom is shown to be proof-theoretically strong even over
${\rm{\Pi }}_1^1$-Comprehension axiom is shown to be proof-theoretically strong even over  ${\rm{RCA}}_0^{\rm{*}}$, and we calibrate the proof-theoretic ordinals of weak fragments of the theory
${\rm{RCA}}_0^{\rm{*}}$, and we calibrate the proof-theoretic ordinals of weak fragments of the theory 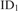 ${\rm{I}}{{\rm{D}}_1}$ of positive inductive definitions over natural numbers. Conjunctions of negative and positive formulas in the transfinite induction axiom of
${\rm{I}}{{\rm{D}}_1}$ of positive inductive definitions over natural numbers. Conjunctions of negative and positive formulas in the transfinite induction axiom of  ${\rm{I}}{{\rm{D}}_1}$ are shown to be weak, and disjunctions are strong. Thus we draw a boundary line between predicatively reducible and impredicative fragments of
${\rm{I}}{{\rm{D}}_1}$ are shown to be weak, and disjunctions are strong. Thus we draw a boundary line between predicatively reducible and impredicative fragments of 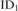 ${\rm{I}}{{\rm{D}}_1}$.
${\rm{I}}{{\rm{D}}_1}$.