Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-29T19:21:07.819Z Has data issue: false hasContentIssue false
  • English
  • Français

On o-minimal expansions of Archimedean ordered groups

Published online by Cambridge University Press:  12 March 2014

Michael C. Laskowski  and
Charles Steinhorn
Michael C. Laskowski
Affiliation:
Department of Mathematics, University of Maryland, College Park, Maryland 20742, E-mail: [email protected]
Charles Steinhorn
Affiliation:
Department of Mathematics, Vassar College, Poughkeepsie, New York 12601, E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We study o-minimal expansions of Archimedean totally ordered groups. We first prove that any such expansion must be elementarily embeddable via a unique (provided some nonzero element is 0-definable) elementary embedding into a unique o-minimal expansion of the additive ordered group of real numbers 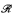. We then show that a definable function in an o-minimal expansion of 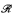enjoys good differentiability properties and use this to prove that an Archimedean real closed field is definable in any nonsemilinear expansion of 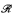. Combining these results, we obtain several restrictions on possible o-minimal expansions of arbitrary Archimedean ordered groups and in particular of the rational ordered group.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 60 , Issue 3 , September 1995 , pp. 817 - 831
Copyright
Copyright © Association for Symbolic Logic 1995

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[BW]Boas, R. P. Jr., and Widder, D. V., Functions with positive differences, Duke Mathematical Journal, vol. 7 (1940), pp. 496–503.Google Scholar
[D]van den Dries, L., Tame topology and o-minimal structures, preprint.Google Scholar
[DL]van den Dries, L. and Lewenberg, A., T-convexity and tame extensions, this Journal, vol. 60 (1995), pp. 74–102.Google Scholar
[DMM]van den Dries, L., Macintyre, A., and Marker, D., The elementary theory of restricted analytic fields with exponentiation, Annals of Mathematics, ser. 2, vol. 140 (1994), pp. 183–205.CrossRefGoogle Scholar
[KPS]Knight, J. F., Pillay, A., and Steinhorn, C., Definable sets in ordered structures. II, Transactions of the American Mathematical Society, vol. 295 (1986), pp. 593–605.CrossRefGoogle Scholar
[L]Lang, S., Algebra, Addison-Wesley, Reading, Massachusetts, 1965.Google Scholar
[M]Marker, D., Omitting types in o-minimal structures, this Journal, vol. 51 (1986), pp. 63–74.Google Scholar
[M2]Marker, D., A strongly minimal expansion of (ω,s), this Journal, vol. 52 (1987), pp. 205–207.Google Scholar
[MPP]Marker, D., Peterzil, Y., and Pillay, A., Additive reducts of real closed fields, this Journal, vol. 57 (1992), pp. 109–117.Google Scholar
[Mi]Miller, C., Exponentiation is hard to avoid, Proceedings of the American Mathematical Society, vol. 122 (1994), pp. 257–259.CrossRefGoogle Scholar
[P]Peterzil, Y., Reducts of some structures over the reals, this Journal, vol. 58 (1993), pp. 955–966.Google Scholar
[PS]Pillay, A. and Steinhorn, C., Definable sets in ordered structures. I, Transactions of the American Mathematical Society, vol. 295 (1986), pp. 565–592.CrossRefGoogle Scholar
[PS2]Pillay, A. and Steinhorn, C., Dedekind complete o-minimal structures, this Journal, vol. 52 (1987), pp. 156–164.Google Scholar
[PS3]Pillay, A. and Steinhorn, C., On discrete o-minimal structures, Annals of Pure and Applied Logic, vol. 34 (1989), pp. 871–885.Google Scholar
[PSS]Pillay, A., Scowcroft, P., and Steinhorn, C., Between groups and rings, Rocky Mountain Journal of Mathematics, vol 9 (1987), pp. 275–289.Google Scholar
[Po]Poston, R. J., Defining multiplication in o-minimal expansions of the additive reals, this Journal (to appear).Google Scholar
[W]Wilkie, A., Model completeness results for expansions of the ordered field of real numbers by restricted Pfaffian functions and the exponential function, Journal of the American Mathematical Society (to appear).Google Scholar