No CrossRef data available.
Published online by Cambridge University Press: 06 January 2025
In [15] we defined and proved the consistency of the principle 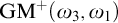 $\mathrm {GM}^+(\omega _3,\omega _1)$ which implies that many consequences of strong forcing axioms hold simultaneously at
$\mathrm {GM}^+(\omega _3,\omega _1)$ which implies that many consequences of strong forcing axioms hold simultaneously at 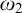 $\omega _2$ and
$\omega _2$ and  $\omega _3$. In this paper we formulate a strengthening of
$\omega _3$. In this paper we formulate a strengthening of 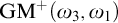 $\mathrm {GM}^+(\omega _3,\omega _1)$ that we call
$\mathrm {GM}^+(\omega _3,\omega _1)$ that we call 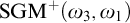 $\mathrm {SGM}^+(\omega _3,\omega _1)$. We also prove, modulo the consistency of two supercompact cardinals, that
$\mathrm {SGM}^+(\omega _3,\omega _1)$. We also prove, modulo the consistency of two supercompact cardinals, that  $\mathrm {SGM}^+(\omega _3,\omega _1)$ is consistent with ZFC. In addition to all the consequences of
$\mathrm {SGM}^+(\omega _3,\omega _1)$ is consistent with ZFC. In addition to all the consequences of 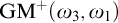 $\mathrm {GM}^+(\omega _3,\omega _1)$, the principle
$\mathrm {GM}^+(\omega _3,\omega _1)$, the principle 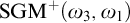 $\mathrm {SGM}^+(\omega _3,\omega _1)$, together with some mild cardinal arithmetic assumptions that hold in our model, implies that any forcing that adds a new subset of
$\mathrm {SGM}^+(\omega _3,\omega _1)$, together with some mild cardinal arithmetic assumptions that hold in our model, implies that any forcing that adds a new subset of 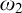 $\omega _2$ either adds a real or collapses some cardinal. This gives a partial answer to a question of Abraham [1] and extends a previous result of Todorčević [16] in this direction.
$\omega _2$ either adds a real or collapses some cardinal. This gives a partial answer to a question of Abraham [1] and extends a previous result of Todorčević [16] in this direction.