Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-25T00:21:14.357Z Has data issue: false hasContentIssue false
  • English
  • Français

Notions of compactness for special subsets of ℝI and some weak forms of the axiom of choice

Published online by Cambridge University Press:  12 March 2014

Marianne Morillon
Marianne Morillon*
Affiliation:
Université de la Réunion, Parc Technologique Universitaire, Ermit, Département de Mathématiques et Informatique, Bâtiment 2, 2 Rue Joseph Wetzell, 97490 Sainte-Clotilde, France, E-mail: [email protected], URL: http://personnel.univ-reunion.fr/mar
Get access Rights & Permissions [Opens in a new window]

Abstract

We work in set-theory without choice ZF . A set is countable if it is finite or equipotent with ℕ. Given a closed subset F of [0, 1]I which is a bounded subset of ℓ 1(I) (resp. such that F ⊆ c 0(I)), we show that the countable axiom of choice for finite sets, (resp. the countable axiom of choice AC ℕ) implies that F is compact. This enhances previous results where AC ℕ (resp. the axiom of Dependent Choices) was required. If I is linearly orderable (for example I = ℝ), then, in ZF , the closed unit ball of the Hilbert space ℓ 2 (I) is (Loeb-)compact in the weak topology. However, the weak compactness of the closed unit ball of 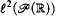is not provable in ZF .

Keywords

Axiom of Choiceproduct topologycompactnesssequential compactness
Type
Research Article
Information
The Journal of Symbolic Logic , Volume 75 , Issue 1 , March 2010 , pp. 255 - 268
Copyright
Copyright © Association for Symbolic Logic 2010

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Avilés, A., The unit ball of the Hilbert space in its weak topology, Proceedings of the American Mathematical Society, vol. 135 (2007), no. 3, pp. 833–836.Google Scholar
[2] Benyamini, Y., Rudin, M. E., and Wage, M., Continuous images of weakly compact subsets of Banach spaces, Pacific Journal of Mathematics, vol. 70 (1977), no. 2, pp. 309–324.Google Scholar
[3] Brunner, N., Products of compact spaces in the least permutation model, Zeitschrift für Mathematische Logik und Grundlagen der Mathematik, vol. 31 (1985), no. 5, pp. 441–448.Google Scholar
[4] Fossy, J. and Morillon, M., The Baire category property and some notions of compactness, Journal of the London Mathematical Society, II, vol. 57 (1998), no. 1, pp. 1–19.Google Scholar
[5] Howard, P., Keremedis, K., Rubin, J. E., and Stanley, A., Compactness in countable Tychonoff products and choice, Mathematical Logic Quarterly, vol. 46 (2000), no. 1, pp. 3–16.Google Scholar
[6] Howard, P. and Rubin, J. E., Consequences of the Axiom of Choice, vol. 59, American Mathematical Society, Providence, RI, 1998.Google Scholar
[7] Jech, T. J., The Axiom of Choice, North-Holland Publishing, Amsterdam, 1973.Google Scholar
[8] Kelley, J. L., The Tychonoff product theorem implies the Axiom of Choice, Fundamenta Mathematicae, vol. 37 (1950), pp. 75–76.Google Scholar
[9] Keremedis, K., The compactness of 2R and the Axiom of Choice, Mathematical Logic Quarterly, vol. 46 (2000), no. 4, pp. 569–571.Google Scholar
[10] Keremedis, K., Tychonoff products of two-element sets and some weakenings of the Boolean prime ideal theorem, Bulletin of the Polish Academy of Sciences Mathematics, vol. 53 (2005), no. 4, pp. 349–359.Google Scholar
[11] Keremedis, K. and Tachtsis, E., On Loeb and weakly Loeb Hausdorff spaces, Scientiae Mathematicae Japonicae, vol. 53 (2001), no. 2, pp. 247–251.Google Scholar
[12] Loeb, P. A., A new proof of the Tychonoff theorem, American Mathematical Monthly, vol. 72 (1965), pp. 711–717.Google Scholar
[13] Morillon, M., Countable choice and compactness, Topology andits Applications, vol. 155 (2008), no. 10, pp. 1077–1088.Google Scholar
[14] Simon, P., On continuous images of Eberlein compacts, Commentationes Mathematicae Universitatis Carolinae, vol. 17 (1976), no. 1, pp. 179–194.Google Scholar