Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Baro, Elías
2011.
on the o-minimal LS-category.
Israel Journal of Mathematics,
Vol. 185,
Issue. 1,
p.
61.
Shiota, Masahiro
2014.
O-minimal Hauptvermutung for polyhedra I.
Inventiones mathematicae,
Vol. 196,
Issue. 1,
p.
163.
Achille, Alessandro
and
Berarducci, Alessandro
2018.
A Vietoris–Smale mapping theorem for the homotopy of hyperdefinable sets.
Selecta Mathematica,
Vol. 24,
Issue. 4,
p.
3445.
Wehrung, Friedrich
2022.
Real spectra and ℓ-spectra of algebras and vector lattices over countable fields.
Journal of Pure and Applied Algebra,
Vol. 226,
Issue. 4,
p.
106861.


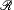 be an o-minimal structure over a real closed field
be an o-minimal structure over a real closed field 