Hostname: page-component-6587cd75c8-gglxz Total loading time: 0.001 Render date: 2025-04-23T23:37:30.039Z Has data issue: false hasContentIssue false
  • English
  • Français

Larger cardinals in Cichoń's diagram

Published online by Cambridge University Press:  12 March 2014

Jörg Brendle
Jörg Brendle*
Affiliation:
Department of Mathematics and Computer Science, Dartmouth College, Hanover, New Hampshire 03755 Mathematisches Institut, Universität Tübingen, W-7400 Tübingen, Germany
Get access Rights & Permissions [Opens in a new window]

Abstract

We prove that in many situations it is consistent with ZFC that part of the invariants involved in Cichoń's diagram are equal to κ while the others are equal to λ, where κ < λ are both arbitrary regular uncountable cardinals. We extend some of these results to the case when λ is singular. We also show that cf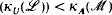 is consistent with ZFC.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 56 , Issue 3 , September 1991 , pp. 795 - 810
Copyright
Copyright © Association for Symbolic Logic 1991

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

REFERENCES

[Ba1] Bartoszyński, T., Additivity of measure implies additivity of category, Transactions of the American Mathematical Society, vol. 281 (1984), pp. 209–213.Google Scholar
[Ba2] Bartoszyński, T., Combinatorial aspects of measure and category, Fundamenta Mathematicae, vol. 127 (1987), pp. 225–239.CrossRefGoogle Scholar
[BJ1] Bartoszyński, T. and Ihoda, J. [Judah, H.], On the cofinality of the smallest covering of the real line by meager sets, this Journal, vol. 54 (1989), pp. 828–832.Google Scholar
[BJ2] Bartoszyński, T. and Ihoda, J., Jumping with random reals (to appear).Google Scholar
[BJS1] Bartoszyński, T., Ihoda, J. [Judah, H.], and Shelah, S., The cofinality of cardinal invariants related to measure and category, this Journal, vol. 54 (1989), pp. 719–726.Google Scholar
[BJS2] Bartoszyński, T., Ihoda, J. [Judah, H.], The Cichon diagram (to appear).Google Scholar
[JS1] Judah, H. and Shelah, S., The Kunen-Miller chart (Lebesgue measure, the Baire property, Laver reals and preservation theorems for forcing), this Journal, vol. 55 (1990), pp. 909–927.Google Scholar
[JS2] Judah, H. and Shelah, S., Around random algebra (to appear).Google Scholar
[Ka] Kamburelis, A., Iterations of Boolean algebras with measure, Archive for Mathematical Logic, vol. 29 (1989), pp. 21–28.CrossRefGoogle Scholar
[Ku1] Kunen, K., Set theory, North-Holland, Amsterdam, 1980.Google Scholar
[Ku2] Kunen, K., Random and Cohen reals, Handbook of set-theoretic topology, North-Holland, Amsterdam, 1984, pp. 887–911.CrossRefGoogle Scholar
[Mil] Miller, A., Some properties of measure and category, Transactions of the American Mathematical Society, vol. 266 (1981), pp. 93–114.CrossRefGoogle Scholar
[Mi2] Miller, A., Additivity of measure implies dominating reals, Proceedings of the American Mathematical Society, vol. 91 (1984), pp. 111–117.CrossRefGoogle Scholar
[RS] Raisonnier, J. and Stern, J., The strength of measurability hypotheses, Israel Journal of Mathematics, vol. 50 (1985), pp. 337–349.CrossRefGoogle Scholar
[T] Truss, J., Sets having calibre ℵ1 , Logic Colloquium '76, North-Holland, Amsterdam, 1977, pp. 595–612.Google Scholar