Hostname: page-component-6bf8c574d5-xtvcr Total loading time: 0 Render date: 2025-02-19T23:02:56.487Z Has data issue: false hasContentIssue false
  • English
  • Français

INDESTRUCTIBILITY OF THE TREE PROPERTY

Published online by Cambridge University Press:  16 September 2019

RADEK HONZIK  and
ŠÁRKA STEJSKALOVÁ
RADEK HONZIK
Affiliation:
DEPARTMENT OF LOGIC CHARLES UNIVERSITY CELETNÁ 20, PRAHA 1 116 42, CZECH REPUBLIC E-mail: [email protected]: logika.ff.cuni.cz/radekE-mail: [email protected]: logika.ff.cuni.cz/sarka
ŠÁRKA STEJSKALOVÁ
Affiliation:
DEPARTMENT OF LOGIC CHARLES UNIVERSITY CELETNÁ 20, PRAHA 1 116 42, CZECH REPUBLIC E-mail: [email protected]: logika.ff.cuni.cz/radekE-mail: [email protected]: logika.ff.cuni.cz/sarka
Get access Rights & Permissions [Opens in a new window]

Abstract

In the first part of the article, we show that if 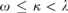$\omega \le \kappa < \lambda$ are cardinals, ${\kappa ^{ < \kappa }} = \kappa$, and λ is weakly compact, then in 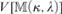$V\left[M {\left( {\kappa ,\lambda } \right)} \right]$ the tree property at $$\lambda = \left( {\kappa ^{ + + } } \right)^{V\left[ {\left( {\kappa ,\lambda } \right)} \right]} $$ is indestructible under all 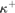${\kappa ^ + }$-cc forcing notions which live in 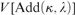$V\left[ {{\rm{Add}}\left( {\kappa ,\lambda } \right)} \right]$, where 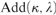${\rm{Add}}\left( {\kappa ,\lambda } \right)$ is the Cohen forcing for adding λ-many subsets of κ and $\left( {\kappa ,\lambda } \right)$ is the standard Mitchell forcing for obtaining the tree property at 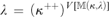$\lambda = \left( {\kappa ^{ + + } } \right)^{V\left[ {\left( {\kappa ,\lambda } \right)} \right]} $. This result has direct applications to Prikry-type forcing notions and generalized cardinal invariants. In the second part, we assume that λ is supercompact and generalize the construction and obtain a model ${V^{\rm{*}}}$, a generic extension of V, in which the tree property at 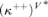${\left( {{\kappa ^{ + + }}} \right)^{{V^{\rm{*}}}}}$ is indestructible under all 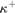${\kappa ^ + }$-cc forcing notions living in 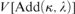$V\left[ {{\rm{Add}}\left( {\kappa ,\lambda } \right)} \right]$, and in addition under all forcing notions living in 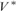${V^{\rm{*}}}$ which are 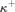${\kappa ^ + }$-closed and “liftable” in a prescribed sense (such as 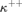${\kappa ^{ + + }}$-directed closed forcings or well-met forcings which are ${\kappa ^{ + + }}$-closed with the greatest lower bounds).

Keywords

03E5503E35tree propertylifting of embeddingsindestructibility
Type
Articles
Information
The Journal of Symbolic Logic , Volume 85 , Issue 1 , March 2020 , pp. 467 - 485
Copyright
Copyright © The Association for Symbolic Logic 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abraham, U., Aronszajn trees on ${\aleph _2}$and .${\aleph _3}$. Annals of Pure and Applied Logic, vol. 24 (1983), no. 3, pp. 213–230.CrossRefGoogle Scholar
Baumgartner, J. E., Iterated forcing, Surveys in Set Theory (Mathias, A. R. D., editor), London Mathematical Society Lecture Note series, vol. 87, Cambridge University Press, Cambridge, 1983, pp. 1–59.Google Scholar
Brooke-Taylor, A., Fischer, V., Friedman, S.-D., and Montoya, D. C., Cardinal characteristics at κ in a small $u\left( \kappa \right)$ model. Annals of Pure and Applied Logic, vol. 168 (2017), no. 1, pp. 37–49.CrossRefGoogle Scholar
Cummings, J., Iterated forcing and elementary embeddings, Handbook of Set Theory, vol. 2 (Foreman, M. and Kanamori, A., editors), Springer, New York, 2010, pp. 775–884.CrossRefGoogle Scholar
Cummings, J. and Foreman, M., The tree property. Advances in Mathematics, vol. 133 (1998), no. 1, pp. 1–32.CrossRefGoogle Scholar
Cummings, J., Friedman, S.-D., Magidor, M., Rinot, A., and Sinapova, D., The eightfold way, this Journal, vol. 83 (2018), no. 1, pp. 349–371.Google Scholar
Džamonja, M. and Shelah, S., Universal graphs at the successor of a singular cardinal, this Journal, vol. 68 (2003), no. 2, pp. 366–388.Google Scholar
Friedman, S.-D., Honzik, R., and Stejskalová, Š., The tree property at ${\aleph _{\omega + 2}}$with a finite gap . Fundamenta Mathematicae, to appear, 2019.Google Scholar
Friedman, S.-D., Honzik, R., and Stejskalová, Š., The tree property at the double sucessor of a singular cardinal with a larger gap. Annals of Pure and Applied Logic, vol. 169 (2018), pp. 548–564.CrossRefGoogle Scholar
Garti, S. and Shelah, S., A strong polarized relation, this Journal, vol. 77 (2012), no. 3, pp. 766–776.Google Scholar
Garti, S. and Shelah, S., The ultrafilter number for singular cardinals. Acta Mathematica Hungarica, vol. 137 (2012), no. 4, pp. 296–301.CrossRefGoogle Scholar
Golshani, M. and Poveda, A., The tree property at double successors of singular cardinals of uncountable cofinality with infinite gaps, preprint, 2019, arXiv:1808.06390.CrossRefGoogle Scholar
Hayut, Y. and Magidor, M., Destructibility of the tree property at ${\aleph _{\omega + 1}}$., this Journal, vol. 84 (2019), no. 2, pp. 621–631.Google Scholar
Honzik, R. and Stejskalová, Š Small $u\left( \kappa \right)$ at singular κ with compactness at ,${\kappa ^{ + + }}$. submitted, 2019.Google Scholar
Jech, T., Set Theory, Springer Monographs in Mathematics, Springer, Berlin, 2003.Google Scholar
Jensen, R. B., Subcomplete forcing and ${\cal L}$-forcing, .${\cal L}$-Forcing, E-Recursion, Forcing and ${{\rm{C}}^{\rm{*}}}$-Algebras (Chong, C., Feng, Q., Slaman, T. A., Hugh Woodin, W., and Yang, Y., editors), Lecture Notes Series, Institute for Mathematical Sciences, National University of Singapore, World Scientific, 2014, pp. 83–182.CrossRefGoogle Scholar
Jensen, R. and Schlechta, K., Result on the generic Kurepa hypothesis. Archive for Mathematical Logic, vol. 30 (1990), pp. 13–27.CrossRefGoogle Scholar
Kanamori, A., Perfect-set forcing for uncountable cardinals. Annals of Mathematical Logic, vol. 19 (1980), pp. 97–114.CrossRefGoogle Scholar
Kunen, K., Saturated ideals, this Journal, vol. 43 (1978), no. 1.CrossRefGoogle Scholar
Kunen, K., Set Theory (Studies in Logic: Mathematical Logic and Foundations), College Publications, London, 2011, pp. 65–76.Google Scholar
Magidor, M., Changing cofinality of cardinals. Fundamenta Mathematicae, vol. 99 (1978), pp. 61–71.CrossRefGoogle Scholar
Neeman, I., The tree property up to ${\aleph _{\omega + 1}}$., this Journal, vol. 79 (2014), no. 1, pp. 429–459.Google Scholar
Shelah, S., Proper and Improper Forcing, second ed., Cambridge University Press, Cambridge, 2016.CrossRefGoogle Scholar
Unger, S., Fragility and indestructibility of the tree property. Archive for Mathematical Logic, vol. 51 (2012), no. 5–6, pp. 635–645.CrossRefGoogle Scholar