Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-22T17:36:15.123Z Has data issue: false hasContentIssue false
  • English
  • Français

Game sentences, recursive saturation and definability

Published online by Cambridge University Press:  12 March 2014

Victor Harnik
Victor Harnik*
Affiliation:
University of Haifa, Mt. Carmel, Haifa, Israel
Get access Rights & Permissions [Opens in a new window]

Extract

In the past 10 years much progress occurred in the direction of unifying the treatment of finitary Lωω and infinitary logic. We have especially in mind the study of countable admissible fragments (of which Lωω is a particular case) initiated by Barwise in [1] (cf. also [2]), the introduction of -recursively saturated structures by Ressayre in [22] (for 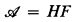these were independently introduced in [3]) and game sentences as studied by Vaught in [26] (see also Svenonius [25] and Moschovakis [19], [20]). A good reference for these topics is [17].

In this paper we illustrate the use of these tools for deducing definability theorems. We go beyond the known results as seen from the discussion that follows.

Let L be a language and P a (say, unary) additional predicate. Consider a sentence σ(P) in the language L(P). There are two types of definability theorems: the “Beth type” and the “Svenonius type”. Beth's theorem [4] characterizes the condition:

(i)′ for any ;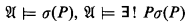

while Svenonius' result [24] characterizes:

(i)″ for any 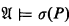, every L-automorphism of is an L(P)-automorphism.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 45 , Issue 1 , March 1980 , pp. 35 - 46
Copyright
Copyright © Association for Symbolic Logic 1980

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Barwise, J., Infinitary logic and admissible sets, this Journal, vol. 34 (1969), pp. 226–252.Google Scholar
[2]Barwise, J., Admissible sets and structures, Springer-Verlag, Berlin and New York, 1975.CrossRefGoogle Scholar
[3]Barwise, J. and Schlipf, J., An introduction to recursively saturated and resplendent models, this Journal, vol. 41 (1976), pp. 531–536.Google Scholar
[4]Beth, E. W., On Padoa's method in the theory of definition, Iniagationes Mathematicae, vol. 15 (1953), pp. 330–339.Google Scholar
[5]Chang, C. C., Some new results in definability, Bulletin of the American Mathematical Society, vol. 70 (1964), pp. 808–813.CrossRefGoogle Scholar
[6]Chang, C. C. and Keisler, H. J., Model theory, North-Holland, Amsterdam, 1973.Google Scholar
[7]Garland, S. J., Generalized interpolation theorems, this Journal, vol. 37 (1972), 343–351.Google Scholar
[8]Harnik, V., Refinements of Vaught's normal form theorem, this Journal vol. 44 (1979), pp. 289–306.Google Scholar
[9]Harnik, V. and Makkai, M., Applications of Vaught sentences and the covering theorem, this Journal, vol. 41 (1976), pp. 171–187.Google Scholar
[10]Harrison, J., Recursive pseudo-wellorderings, Ph.D. Thesis, Stanford University, 1966.Google Scholar
[11]Keisler, H. J., Model theory for infinitary logic, North-Holland, Amsterdam, 1971.Google Scholar
[12]Lopez-Escobar, E. G. K., An interpolation theorem for denumerably long sentences, Fundamenta Mathematicae, vol. 57 (1965), pp. 253–272.CrossRefGoogle Scholar
[13]Kueker, D. W., Generalized interpolation and definability, Annals of Mathematical Logic, vol. 1 (1970), pp. 423–468.CrossRefGoogle Scholar
[14]Maehara, S. and Takeuti, G., Two interpolation theorems for a Π11 predicate calculus, this Journal, vol. 36 (1971), pp. 262–270.Google Scholar
[15]Makkai, M., On a generalization of a theorem of E. W. Beth, Acta Mathematica Academiae Scientarium Hungaricae, vol. 15 (1964), pp. 227–235.CrossRefGoogle Scholar
[16]Makkai, M., Global definability theory in Lω1ω, Bulletin of the American Mathematical Society, vol. 79 (1973), pp. 916–921.CrossRefGoogle Scholar
[17]Makkai, M., Admissible sets and infinitary logic, Handbook of mathematical logic (Barwise, J., Editor), North-Holland, Amsterdam, 1977, pp. 233–281.CrossRefGoogle Scholar
[18]Makkai, M., An “admissible” generalization of a theorem on countable Σ11 sets with applications, Annals of Mathematical Logic, vol. 11, pp. 1977–1.Google Scholar
[19]Moschovakis, Y. N., The Suslin-Kleene theorem for countable structures, Duke Mathematical Journal, vol. 37 (1970), pp. 341–352.CrossRefGoogle Scholar
[20]Moschovakis, Y. N., The game quantifier, Proceedings of the American Mathematical Society, vol. 31 (1972), pp. 245–250.CrossRefGoogle Scholar
[21]Motohashi, N., A new theorem on definability, Dissertation, University of Tokyo, 1971.Google Scholar
[22]Ressayre, J.-P., Models with compactness properties relative to an admissible language, Annals of Mathematical Logic, vol. 11 (1977), pp. 31–55.CrossRefGoogle Scholar
[23]Reyes, G.E., Local definability theory, Annals of Mathematical Logic, vol. 1 (1970), pp. 95–137.CrossRefGoogle Scholar
[24]Svenonius, L., A theorem on permutations in models, Theoria vol. 25 (1959), pp. 173–178.CrossRefGoogle Scholar
[25]Svenonius, L., On the denumerable models of theories with extra predicates, The theory of models, North-Holland, Amsterdam, 1965, pp. 376–389.Google Scholar
[26]Vaught, R.L., Descriptive set theory in Lω1ω, Lecture Notes in Mathematics no. 337, Cambridge Summer School in Mathematical Logic, Springer, New York, 1973, pp. 574–598.Google Scholar
[27]Keisler, H.J., Finite approximations of infinitely long formulas, The theory of models, North-Holland, Amsterdam, 1965, pp. 158–169.Google Scholar