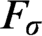Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-29T01:12:35.872Z Has data issue: false hasContentIssue false
  • English
  • Français

$F_\sigma $ GAMES AND REFLECTION IN 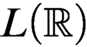$L(\mathbb {R})$

Part of: General logic Computability and recursion theory Set theory

Published online by Cambridge University Press:  21 July 2020

J. P. AGUILERA
J. P. AGUILERA*
Affiliation:
DEPARTMENT OF MATHEMATICS UNIVERSITY OF GHENT KRIJGSLAAN 281-S8, 9000GHENT, BELGIUM INSTITUTE OF DISCRETE MATHEMATICS AND GEOMETRY VIENNA UNIVERSITY OF TECHNOLOGY WIEDNER HAUPTSTRAßE 8-10, 1040VIENNA, AUSTRIAE-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

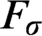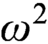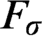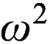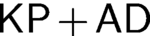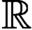We characterize the determinacy of $F_\sigma $ games of length $\omega ^2$ in terms of determinacy assertions for short games. Specifically, we show that $F_\sigma $ games of length $\omega ^2$ are determined if, and only if, there is a transitive model of ${\mathsf {KP}}+{\mathsf {AD}}$ containing $\mathbb {R}$ and reflecting 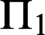$\Pi _1$ facts about the next admissible set.

As a consequence, one obtains that, over the base theory 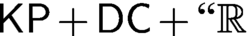${\mathsf {KP}} + {\mathsf {DC}} + ``\mathbb {R}$ exists,” determinacy for $F_\sigma $ games of length $\omega ^2$ is stronger than ${\mathsf {AD}}$ , but weaker than ${\mathsf {AD}} + \Sigma _1$ -separation.

Keywords

03D60F∑ gamesdeterminacylong gamesadmissible setreflecting model

MSC classification

Primary: 03B30: Foundations of classical theories (including reverse mathematics)
Secondary: 03D60: Computability and recursion theory on ordinals, admissible sets, etc. 03D70: Inductive definability 03E15: Descriptive set theory
Type
Articles
Information
The Journal of Symbolic Logic , Volume 85 , Issue 3 , September 2020 , pp. 1102 - 1123
Check for updates
Copyright
© The Association for Symbolic Logic 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Aczel, P. and Richter, W., Inductive definitions and reflecting properties of admissible ordinals, generalized recursion theory , Generalized Recursion Theory: Proceedings of the 1972 Oslo Symposium (Fenstad, J. E. and Hinman, P. G., editors), Studies in Logic and the Foundations of Mathematics, vol. 79, Elsevier, Amsterdam, 1974, pp. 301–381.Google Scholar
Aguilera, J. P., Determined admissible sets . Proceedings of the American Mathematical Society , vol. 148 (2020), pp. 2217–2231.CrossRefGoogle Scholar
Aguilera, J. P., Between the finite and the infinite , Ph.D. thesis, 2019.Google Scholar
Aguilera, J. P., Long Borel games. Israel Journal of Mathematics , forthcoming.Google Scholar
Aguilera, J. P., Shortening clopen games, 2018, forthcoming.Google Scholar
Aguilera, J. P. and Müller, S., The consistency strength of long projective determinacy , this Journal, vol. 85 (2020), no. 1, pp. 338–366.Google Scholar
Barwise, K. J., Gandy, R. O., and Moschovakis, Y. N., The next admissible set , this Journal, vol. 36 (1971), pp. 108–120.Google Scholar
Blass, A., Equivalence of two strong forms of determinacy . Proceedings of the American Mathematical Society , vol. 52 (1975), pp. 373–376.CrossRefGoogle Scholar
Gale, D. and Stewart, F. M., Infinite games with perfect information , Contributions to the Theory of Games , vol. 2 (Kuhn, H. W. and Tucker, A. W., editors), Princeton University Press, Princeton, NJ, 1953, pp. 245–266.Google Scholar
Harrington, L. A. and Kechris, A. S., On monotone vs. nonmonotone induction, handwritten notes dated September 1975. 9 pp.Google Scholar
Harrington, L. A. and Kechris, A. S., On monotone vs. nonmonotone induction . Bulletin of the American Mathematical Society , vol. 82 (1976), pp. 888–890.CrossRefGoogle Scholar
Harrington, L. A. and Moschovakis, Y. N., On positive induction vs. nonmonotone induction, mimeographed notes.Google Scholar
Kechris, A. S., On spector classes , Ordinal Definability and Recursion Theory: The Cabal Seminar (Kechris, A. S., Löwe, B., and Steel, J. R., editors), Lecture Notes in Logic, vol. 3, Cambridge University Press, Cambridge, MA, 2016.CrossRefGoogle Scholar
Kechris, A. S. and Woodin, W. H., Equivalence of partition properties and determinacy . Proceedings of the National Academy of Sciences , vol. 80 (1983), pp. 1783–1786.CrossRefGoogle ScholarPubMed
Koellner, P. and Woodin, W. H., Large cardinals from determinacy , Handbook of Set Theory (Foreman, M. and Kanamori, A., editors), Springer, New York, 2010.Google Scholar
Moschovakis, Y. N., Determinacy and prewellorderings of the continuum , Mathematical Logic and Foundations of Set Theory (Bar-Hillel, Y., editor), North-Holland, Amsterdam, 1970, pp. 24–62.Google Scholar
Moschovakis, Y. N., Uniformization in a playful universe . Bulletin of the American Mathematical Society , vol. 77 (1971), pp. 731–736.CrossRefGoogle Scholar
Moschovakis, Y. N., The game quantifier . Proceedings of the American Mathematical Society , vol. 31 (1972), pp. 245–250.CrossRefGoogle Scholar
Moschovakis, Y. N., Elementary Induction on Abstract Structures , Studies in Logic and the Foundations of Mathematics, Elsevier, 1974.Google Scholar
Moschovakis, Y. N., Descriptive Set Theory , second ed., Mathematical Surveys and Monographs, vol. 155, AMS, New York, 2009.CrossRefGoogle Scholar
Neeman, I., The Determinacy of Long Games , De Gruyter Series in Logic and Its Applications, Walter de Gruyter, Berlin, 2004.CrossRefGoogle Scholar
Steel, J. R., Scales in L(R) , Games, Scales and Suslin Cardinals: The Cabal Seminar (Kechris, A. S., Löwe, B., and Steel, J. R., editors), Lecture Notes in Logic, vol. 1, Cambridge University Press, Cambridge, MA, 2008.Google Scholar
Tanaka, K., Weak axioms of determinacy and subsystems of analysis II . Annals of Pure and Applied Logic , vol. 52 (1991), pp. 181–193.CrossRefGoogle Scholar
Trang, N. D., Generalized solovay measures, the HOD analysis, and the Core model induction , Ph.D. thesis, University of California at Berkeley, 2013.Google Scholar
Uhlenbrock, S., Pure and hybrid mice with finitely many woodin cardinals from levels of determinacy , Ph.D. thesis, WWU Münster, 2016.Google Scholar
Wolfe, P., The strict determinateness of certain infinite games . The Pacific Journal of Mathematics , vol. 5 (1955), pp. 841–847.CrossRefGoogle Scholar