Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-07T07:52:35.771Z Has data issue: false hasContentIssue false
  • English
  • Français

From index sets to randomness in ∅n: random reals and possibly infinite computations part II

Published online by Cambridge University Press:  12 March 2014

Verónica Becher  and
Serge Grigorieff
Verónica Becher
Affiliation:
Departamento de Computación, Facultad de Ciencias Exactas y Naturales, Universidad de Buenos Aires, Conicet, Argentina, E-mail: [email protected]
Serge Grigorieff
Affiliation:
Liafa, Université Paris7 & CNRS, France, E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We obtain a large class of significant examples of n-random reals (i.e., Martin-Löf random in oracle ∅(n−1)) à la Chaitin. Any such real is defined as the probability that a universal monotone Turing machine performing possibly infinite computations on infinite (resp. finite large enough, resp. finite self-delimited) inputs produces an output in a given set 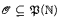. In particular, we develop methods to transfer 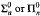many-one completeness results of index sets to n-randomness of associated probabilities.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 74 , Issue 1 , March 2009 , pp. 124 - 156
Copyright
Copyright © Association for Symbolic Logic 2009

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Becher, V. and Chaitin, G., Another example of higher order randomness, Fundamenta Informaticae, vol. 51 (2002), no. 4, pp. 325–338.Google Scholar
[2]Becher, V., Chaitin, G., and Daicz, S., A highly random number, Proceedings of the Third Discrete Mathematics and Theoretical Computer Science Conference (DMTCS'01) (Calude, C.S., Dineen, M.J., and Sburlan, S., editors), Springer-Verlag, 2001, pp. 55–68.Google Scholar
[3]Becher, V., Figueira, S., Grigorieff, S., and Miller, J.S., Randomness and halting probabilities, this Journal, vol. 71 (2006), no. 4, pp. 1411–1430.Google Scholar
[4]Becher, V. and Grigorieff, S., Recursion and topology on 2≤ω for possibly infinite computations, Theoretical Computer Science, vol. 322 (2004), pp. 85–136.CrossRefGoogle Scholar
[5]Becher, V. and Grigorieff, S., Random reals and possibly infinite computations. Part I: Randomness in ∅′, this Journal, vol. 70 (2005), no. 3, pp. 891–913.Google Scholar
[6]Becher, V. and Grigorieff, S., Random reals à la Chaitin with no prefix-freeness, Theoretical Computer Science, vol. 385 (2007), pp. 193–201.CrossRefGoogle Scholar
[7]Becher, V. and Grigorieff, S., Randomness and Outputs in a computable ordered set (Random reals and possibly infinite computations: Part III), (2008), in preparation.Google Scholar
[8]Calude, C.S., Hertling, P.H., and Khoussainov, B.Wang, Y., Recursively enumerable reals and Chaitin Ω numbers, Stacs 98 (Paris, 1998), Lecture Notes in Computer Science, vol. 1373, Springer-Verlag, 1998, pp. 596–606.CrossRefGoogle Scholar
[9]Chaitin, G., A theory of program size formally identical to information theory, Journal of the ACM, vol. 22 (1975), pp. 329–340, Available on Chaitin's home page.CrossRefGoogle Scholar
[10]Downey, R. and Hirschfeldt, D., Algorithmic randomness and complexity, Springer, 2008, to appear.Google Scholar
[11]Hjorth, G. and Nies, A., Randomness via effective descriptive set theory, The Journal of the London Mathematical Society, vol. 75 (2007), no. 2, pp. 495–508.CrossRefGoogle Scholar
[12]Kreisel, G., Shoenfield, J.R., and Wang, H., Number theoretic concepts and recursive well-orderings, Archivfur math. Logik und Grundlagenforschung, vol. 5 (1960), pp. 42–64.CrossRefGoogle Scholar
[13]Rogers, H., Theory of recursive functions and effective computability, McGraw-Hill, 1967.Google Scholar
[14]Sacks, G.E., Degrees of unsolvability, Annals of Mathematical Studies, Princeton University Press, 1966.Google Scholar
[15]Scott, D.S., Continuous lattices, Toposes, algebraic geometry and logic (Lawvered, F.W., editor), Lecture Notes in Math., vol. 2, Springer, 1972, pp. 97–136.CrossRefGoogle Scholar
[16]Selivanov, V.L., Hierarchies in φ-spaces and applications. Mathematical Logic Quaterly, vol. 51 (2005), no. 1, pp. 45–61.CrossRefGoogle Scholar
[17]Soare, R., Recursively enumerable sets and degrees, Perspectives in Mathematical Logic, Springer, 1986.Google Scholar
[18]Stillwell, J., Decidability of the almost all theory of degrees, this Journal, vol. 37 (1972), pp. 501–506.Google Scholar
[19]Wadge, W.W., Degrees of complexity of subsets of the Baire space, Notices of the American Mathematical Society, (1972), pp. A–714.Google Scholar